1) B-metric space
B度量空间
2) B-spline scale space
B-样条尺度空间
1.
Corner detection based on evolution difference of plannar curves in B-spline scale space
B-样条尺度空间轮廓演化差异的角点检测
2.
The multi-scale representation of covariance matrix for planar counter over its Region of Support (ROS) was defined in the framework of B-spline scale space.
在B-样条尺度空间下定义了平面轮廓在其支撑区域(ROS)内的协方差矩阵的多尺度表示,矩阵的最大特征值对应的向量表示轮廓切线方向。
3.
So the main work in this paper is to investigate how to improve the performance of feature detection using different tools such as Determinants of Covariance Matrices, Gradient Correlation Matrices, Laplace transformation in Gaussian scale space and the evolution difference of planar curves in B-spline scale space.
基于此,本文的研究工作主要包括:研究平面曲线协方差矩阵的特征值和性质,在高斯尺度空间构建轮廓曲线的梯度相关矩阵,研究轮廓曲线的拉普拉斯变换和在B-样条尺度空间研究轮廓演化差异等轮廓曲线的新特性和新方法,为此设计几种角点检测算法,并对提出的各种角点检测器进行性能评估。
3) metric space
度量空间
1.
Iterative processes for generalized asymptotically non-expansive mapping in convex metric space;
度量空间中广义渐进非扩张映射Ishikawa迭代的收敛性问题
2.
Discussion on the sets both open and close in the metric space;
度量空间中既开又闭的集合探讨
3.
Chain recurrent points and ω-limiting points in metric space;
度量空间中的链回归点与ω-极限点
4) metric spaces
度量空间
1.
A rectifible property of sets in metric spaces;
关于度量空间中泛流的注记(英文)
2.
In this paper,internal characterizations onthe compact-covering cs-pi images of metric spaces and the compact-covering cs images of locally separable metric spacesare obtained.
给出了度量空间的紧覆盖cs-π映象和局部可分度量空间的紧覆盖cs映象的内在刻画。
3.
Internal Characterizations of CS?mapping images( Compact ? covering CS ? mapping images ) of metric spaces.
分别建立了度量空间在CS 映射和紧复盖CS 映射下的象空间的特征 。
5) spatial measure
空间度量
1.
This paper gives a definition of spatial measure on spatial data cube for multi-source data on "Digital City",and describes basic concept of aggregation on spatial measure,and explains aggregation process of point spatial measure,line spatial measure,area spatial measure by legend,and states basic aggregation principle of spatial measure,and expresses foundatio.
叙述了面向“数字城市”多源数据的空间数据立方体空间度量的基本定义;描述了空间度量的聚集概念,并结合具体的图例阐述了点状、线状、面状空间度量的聚集过程;解释了空间数据立方体维上钻、维下翻、维层次上钻、维层次下翻的空间度量聚集操作基本原理。
6) convex metric space
凸度量空间
1.
New Ishikawa iteration approximation with errors for asymptotically quasi-nonexpansive mappings in convex metric space;
凸度量空间中渐近拟非扩张映象新的带误差的Ishikawa迭代逼近
2.
Convergence of Ishikawa type iterative sequence of asymptoticallyquasi-nonexpansive mappings in convex metric space;
凸度量空间中渐近拟非扩张映象的Ishikawa型迭代序列的收敛性
3.
A fixed point existence theorem and a convergence theorem in convex metric spaces;
完备凸度量空间中不动点定理与收敛定理
补充资料：度量空间
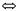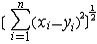| 度量空间 metric space 具有度量的抽象空间，设X是一个集合，若有定义在X×X上的非负实值函数d，满足①d（x，y）≥0，d（x，y）＝0  x＝y； ②d（x，y）＝d（y，x）；③d（x，z）≤d（x，y）＋d（y，z），则称（X，d）是度量空间，d称为距离或度量。这是最接近于欧几里得空间的抽象空间。利用度量可很自然地将欧几里得空间上点的邻域、开集、闭集，收敛序列以及连续映射等概念推广到一般度量空间，也能将一致连续的概念推广到度量空间。由于19世纪末集合论产生后，实变函数及泛函分析的发展，需要规定函数间的距离，因而抽象出度量、度量空间的概念，其创始人是M.R.弗雷歇。常见的度量空间有：n维欧几里得空间（Rn,d）：Rn＝｛（x1，…，xn）｜xi∈R，i＝1，2，…，n ｝，d（x，y）＝  ，其中x＝（x1，x2，…， xn），y＝（y1，y2，…，yn）。希尔 伯特空 间（l2；d）：l2＝｛（x1，x2，…，xn…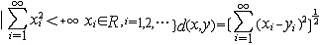）  ， 其中x ＝( x1，x2 ，…），y＝（y1，y2，…）∈l2。函数空间(ρ[0，1］，d)：C[0，1］＝｛f：f为[0，1］上的实值连续函数｝，对任意f，g∈C[0，1］，d(f，g)＝max｛｜f（x）－g（x）｜｝。 x∈[0，1］ 对度量空间（X，d）可引进拓扑结构，即以包含开球B（x，r）＝｛y∈X｜d（ x，y）＜r ｝的集为邻域定义拓扑，称为d所诱导的拓扑。 |
说明：补充资料仅用于学习参考，请勿用于其它任何用途。
参考词条