1) g-metric spaces
g-度量空间
1.
As an application of above results , we prove that almost open, closed mappings preserve metric spaces, g -metric spaces and SB-metric spaces.
作为这一结果的一个应用,本文证明了几乎开,闭映射保持度量空间,g-度量空间,sn-度量空间。
2) g-metrizable spaces
g-可度量空间
1.
In this paper,the relations between metrizable spaces and Ν-spaces or g-metrizable spaces are established by sequence-covering stratified strong compact mappings.
本文利用序列覆盖分层强紧映射,建立了Ν-空间,g-可度量空间与特定的度量空间的关系,这是对Alexandroff的部分问题的肯定回答。
2.
In the second part, the ralations between metrizable spaces and g-metrizable spaces are established by weak-open mapping.
第二部分利用弱开映射，建立了g-可度量空间与度量空间之间的关系，得到了对度量空间弱开k-映射的一些等价刻画并证明了度量空间、g-可度量空间、sn-可度量空间、N-空间在弱开、闭映射下保持。
3) G-convex metric spaces
G-凸度量空间
4) g-metrizable spaces
g可度量空间
5) G unit logical metric space
G单位逻辑度量空间
1.
Based on this concept,it determined a metric ρ,and(,ρ) becomes a metric space(It is called G unit logical metric space).
给出了G单位区间[0,1]的定义并在其上引入了元素间的可分度的概念,讨论了其基本性质,并在此定义的基础上确定了一个度量ρ,从而([0,1],ρ)成为一个度量空间(文中称“G单位逻辑度量空间”),并对G单位逻辑度量空间的性质及其结构进行了详尽的讨论,并得到一些好的结果。
6) metric space
度量空间
1.
Iterative processes for generalized asymptotically non-expansive mapping in convex metric space;
度量空间中广义渐进非扩张映射Ishikawa迭代的收敛性问题
2.
Discussion on the sets both open and close in the metric space;
度量空间中既开又闭的集合探讨
3.
Chain recurrent points and ω-limiting points in metric space;
度量空间中的链回归点与ω-极限点
补充资料：度量空间
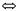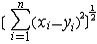| 度量空间 metric space 具有度量的抽象空间，设X是一个集合，若有定义在X×X上的非负实值函数d，满足①d（x，y）≥0，d（x，y）＝0  x＝y； ②d（x，y）＝d（y，x）；③d（x，z）≤d（x，y）＋d（y，z），则称（X，d）是度量空间，d称为距离或度量。这是最接近于欧几里得空间的抽象空间。利用度量可很自然地将欧几里得空间上点的邻域、开集、闭集，收敛序列以及连续映射等概念推广到一般度量空间，也能将一致连续的概念推广到度量空间。由于19世纪末集合论产生后，实变函数及泛函分析的发展，需要规定函数间的距离，因而抽象出度量、度量空间的概念，其创始人是M.R.弗雷歇。常见的度量空间有：n维欧几里得空间（Rn,d）：Rn＝｛（x1，…，xn）｜xi∈R，i＝1，2，…，n ｝，d（x，y）＝  ，其中x＝（x1，x2，…， xn），y＝（y1，y2，…，yn）。希尔 伯特空 间（l2；d）：l2＝｛（x1，x2，…，xn…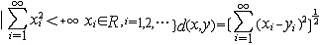）  ， 其中x ＝( x1，x2 ，…），y＝（y1，y2，…）∈l2。函数空间(ρ[0，1］，d)：C[0，1］＝｛f：f为[0，1］上的实值连续函数｝，对任意f，g∈C[0，1］，d(f，g)＝max｛｜f（x）－g（x）｜｝。 x∈[0，1］ 对度量空间（X，d）可引进拓扑结构，即以包含开球B（x，r）＝｛y∈X｜d（ x，y）＜r ｝的集为邻域定义拓扑，称为d所诱导的拓扑。 |
说明：补充资料仅用于学习参考，请勿用于其它任何用途。
参考词条