1) Solution of the cauchy problem of the n-dimension 2-order parabolic equation


二阶抛物型方程柯西问题的解
2) Cauchy problem of parabola equation


抛物型方程的Cauchy问题
3) boundary value problem of parabolic differential equation


抛物型方程的边值问题
4) wave equation of two dimensionality Cauchy question


二维波动方程柯西问题
1.
By using the way of drop dimensionality,this paper works out the integral representation of wave equation of two dimensionality Cauchy question from the viewpoint of Poisson formulas physics explaination and some examples are given to illustrate it.
从泊松公式的物理学意义出发 ,借助降维法推出二维波动方程柯西问题的积分表达式 ,并通过举例给予证
5) two-order parabolic equation


二阶抛物型方程
1.
A family of high-accurate and three-layer difference schemes containing parameters are constructed for solving two-order parabolic equation.
对二阶抛物型方程构造了一族含参数高精度三层差分格式。
6) second-order parabolic problem


二阶抛物问题
1.
Then we simulate the following second-order parabolic problem by least-squares expanded mixed finite method.
其次,本文对二阶抛物问题也提出了相应的最小二乘扩展混合有限元方法。
补充资料:抛物型偏微分方程
| 抛物型偏微分方程 parabolic type,partial differential equation of 偏微分方程的一类。最典型的是热传导方程 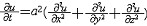 (a>0) (1)基本解是点热源的影响函数。若在t=0时在(ξ,η,ζ)处给定单位点热源,即u0(x0,y0,z0,0)=δ(ξ,η,ζ)(δ为狄拉克函数),则当t>0时便引起在R3的温度分布,这就是基本解。用傅里叶变换可得到它的表达式 (a>0) (1)基本解是点热源的影响函数。若在t=0时在(ξ,η,ζ)处给定单位点热源,即u0(x0,y0,z0,0)=δ(ξ,η,ζ)(δ为狄拉克函数),则当t>0时便引起在R3的温度分布,这就是基本解。用傅里叶变换可得到它的表达式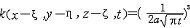 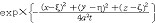 热传导方程初值问题的解可用基本解叠加而成,即 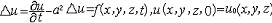 的解为 的解为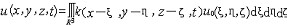 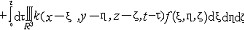 极值原理:一个内部有热源的传导过程,它的最低温度一定在边界上或初始时刻达到。更强的结论是 :如果t=T时在Ω内某一点达到最低温度 ,则在这个时刻以前(t<T时)u≡常数 ;又:若最低温度在t=T时边界¶Ω上某点P达到,则在这点上 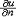 |P,Τ<0(n为外法线方向)。 |P,Τ<0(n为外法线方向)。 |
说明:补充资料仅用于学习参考,请勿用于其它任何用途。
参考词条