1) parabolic equation of higher order


高阶抛物型方程
1.
A three-layer explicit difference scheme is proposed for solving the parabolic equation of higher order [SX(] u[] t[SX)]=(-1) m+1 [SX(] 2m u[] t 2m [SX)] (where m is a positive integer).
对高阶抛物型方程提出一个三层显式差分格式,其局部截断误差阶是O(τ2+h4)。
2.
For solving the parabolic equation of higher order [SX(] u[] t[SX)]=(-1) m+1 [SX(] 2m u[] x 2m [SX)] (where m is a positive integer), a family of three-layered implicit difference schemes containing biparameters are constructed.
对高阶抛物型方程t=(-1)m+1x2m(m为正整数),构造一族含双参数的三层隐式差分格式·在特殊情况下,当参数α=21,β=0时得到一个双层格式·这些格式的截断误差阶均为O((Δt)2+(Δx)4)。
2) high order parabolic equation


高阶抛物型方程
1.
For Solving high order parabolic equation = (?1)m+1 ?t (where m is positive inerger), the author advances a ?x2m two-layer implicit difference scheme with order of local truncation error o(τ + h4) , and when m = 1, 2, 3 , the scheme is proved to 2 be absolutely stable.
本文构造出解高阶抛物型方程=(?1)m+12?t?x2m(m为正整数)的局部截断误差阶为o(τ+h4)的两层隐式差分格式,并证明了当m=1,2,3是它是绝对稳定的。
3) Higher-order parabolic system


高阶抛物型方程组
5) four order parabolic equations


四阶抛物型方程
1.
A two-level explicit finite difference scheme of high accuracy for solving four order parabolic equations is presented,the stability condition of the presented scheme is r=τ/h4≤264/3601 and the truncation order iso(τ2+h8).
给出了一个求解四阶抛物型方程高精度两层显式差分格式,证明了其截断误差为O(τ2+h8),稳定性条件为r=τ/h4≤264/3601。
2.
A ten-point two-level explicit finite difference scheme to solve four order parabolic equations is presented,and it is demonstrated that the stability condition of the presented scheme is ο(τ2+h4) and the truncation order is r=τh4≤79384.
给出了一个求解四阶抛物型方程的两层十点显式差分格式,证明了其截断误差为ο(τ2+h4),稳定性条件为r=hτ4≤37894。
6) four order parabolic equation


四阶抛物型方程
1.
At present,some researchers have got a lot of good numerical solutions for the periodic initial value problem of four order parabolic equation: such as finite difference method,finite elements method,spectral Galerkin method and so on.
本文首先将四阶抛物型方程转化为一个二阶的偏微分方程组,然后对时间项采用子域精细积分的方法、空间项采用三次样条基本公式进行离散,得到了一个含参数α>0(αh)的无条件稳定的差分格式,所得到的差分方程为五点、两层隐格式,它的局部截断误差为O(2τ+α2τ+h4)。
2.
To solve four order parabolic equation,a class of three-layer implicit different schemes containing double parameters are constructed.
对四阶抛物型方程构造一族新的含双参数三层隐式差分格式,并证明该族格式对任意非负参数都是绝对稳定,并且其局部截断误差达到O[(Δt)2+(Δx)8],通过数值例子表明该格式是有效的。
3.
For solving four order parabolic equation ut+ 4ux 4=0, the author advances two new classes of three layered implicit difference scheme with coefficient matrix of tridiagonal type.
为了解四阶抛物型方程 u t+ 4u x4=0 ,建立两类新的、具三对角线型系数矩阵的三层隐式差分格式 其局部截断误差阶均为O(τ2 +h2 +(τh) 2 ) ,且都是绝对稳定的 ,并可用追赶法容易地求解 数值例子表明这些格式是有效
补充资料:抛物型偏微分方程
| 抛物型偏微分方程 parabolic type,partial differential equation of 偏微分方程的一类。最典型的是热传导方程 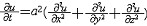 (a>0) (1)基本解是点热源的影响函数。若在t=0时在(ξ,η,ζ)处给定单位点热源,即u0(x0,y0,z0,0)=δ(ξ,η,ζ)(δ为狄拉克函数),则当t>0时便引起在R3的温度分布,这就是基本解。用傅里叶变换可得到它的表达式 (a>0) (1)基本解是点热源的影响函数。若在t=0时在(ξ,η,ζ)处给定单位点热源,即u0(x0,y0,z0,0)=δ(ξ,η,ζ)(δ为狄拉克函数),则当t>0时便引起在R3的温度分布,这就是基本解。用傅里叶变换可得到它的表达式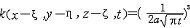 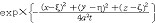 热传导方程初值问题的解可用基本解叠加而成,即 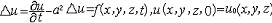 的解为 的解为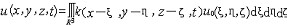 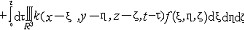 极值原理:一个内部有热源的传导过程,它的最低温度一定在边界上或初始时刻达到。更强的结论是 :如果t=T时在Ω内某一点达到最低温度 ,则在这个时刻以前(t<T时)u≡常数 ;又:若最低温度在t=T时边界¶Ω上某点P达到,则在这点上 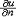 |P,Τ<0(n为外法线方向)。 |P,Τ<0(n为外法线方向)。 |
说明:补充资料仅用于学习参考,请勿用于其它任何用途。
参考词条