1) fourth order parabolic equations


四阶抛物方程
1.
Parallel alternating group explicit schemes for fourth order parabolic equations;


四阶抛物方程——一类新的交替分组显格式
2.
The Finite Difference Parallel Algorithms for Fourth Order Parabolic Equations;


四阶抛物方程有限差分并行算法
3.
A group of Saul yev asymmetric difference schemes for approach the fourth order parabolic equations is given in this paper.
给出了逼近四阶抛物方程的一组新Saul’yev非对称差分格式,利用这组非对称格式和对称的Crank-N icolson格式构造了一类新的并行交替分段隐格式算法,并证明了该算法的绝对稳定性。
2) fourth order parabolic equation


四阶抛物方程
1.
Next, the bicubic Hermite element is applied to another fourth order parabolic equation on an.
本文在各向异性网格下,首先把非协调的ACM元应用于四阶抛物方程的半离散格式,通过高精度分析技巧得到了超逼近性质,进而通过适当的插值后处理技术得到了整体超收敛结果。
2.
We investigate a fourth order parabolic equation with nonnegative large initial value and Dirichlet-Neumann boundary condition in this paper.
本文研究一个四阶抛物方程的非负大初值混合Dirichlet-Neumann边值问题。
3) the parabolic equation of fourth order


四阶抛物方程
1.
Recently, the parabolic equation of fourth order has been caused research interest due to its significant application in the study of physical phenomena in modern applied science, for instance, phase transitions (Cahn-Hilliard equation), the diffusion process of droplet on the solid surface (thin film equation), electric charge transportation of semiconductor (QHD), and so on (see[2,3]).
最近,四阶抛物方程因其在现代应用科学中的重要应用,如用于研究相变的Cahn-Hilliard方程,描述固体表面微滴的扩散过程的薄膜(thin film)方程,及模拟半导体电荷运输的量子流体力学(quantum hydrodynamics)方程(参见文献[2,3])等,而被引起广泛关注和研究兴趣。
4) four order parabolic equations


四阶抛物型方程
1.
A two-level explicit finite difference scheme of high accuracy for solving four order parabolic equations is presented,the stability condition of the presented scheme is r=τ/h4≤264/3601 and the truncation order iso(τ2+h8).
给出了一个求解四阶抛物型方程高精度两层显式差分格式,证明了其截断误差为O(τ2+h8),稳定性条件为r=τ/h4≤264/3601。
2.
A ten-point two-level explicit finite difference scheme to solve four order parabolic equations is presented,and it is demonstrated that the stability condition of the presented scheme is ο(τ2+h4) and the truncation order is r=τh4≤79384.
给出了一个求解四阶抛物型方程的两层十点显式差分格式,证明了其截断误差为ο(τ2+h4),稳定性条件为r=hτ4≤37894。
5) four order parabolic equation


四阶抛物型方程
1.
At present,some researchers have got a lot of good numerical solutions for the periodic initial value problem of four order parabolic equation: such as finite difference method,finite elements method,spectral Galerkin method and so on.
本文首先将四阶抛物型方程转化为一个二阶的偏微分方程组,然后对时间项采用子域精细积分的方法、空间项采用三次样条基本公式进行离散,得到了一个含参数α>0(αh)的无条件稳定的差分格式,所得到的差分方程为五点、两层隐格式,它的局部截断误差为O(2τ+α2τ+h4)。
2.
To solve four order parabolic equation,a class of three-layer implicit different schemes containing double parameters are constructed.
对四阶抛物型方程构造一族新的含双参数三层隐式差分格式,并证明该族格式对任意非负参数都是绝对稳定,并且其局部截断误差达到O[(Δt)2+(Δx)8],通过数值例子表明该格式是有效的。
3.
For solving four order parabolic equation ut+ 4ux 4=0, the author advances two new classes of three layered implicit difference scheme with coefficient matrix of tridiagonal type.
为了解四阶抛物型方程 u t+ 4u x4=0 ,建立两类新的、具三对角线型系数矩阵的三层隐式差分格式 其局部截断误差阶均为O(τ2 +h2 +(τh) 2 ) ,且都是绝对稳定的 ,并可用追赶法容易地求解 数值例子表明这些格式是有效
6) fourth order parabolic equation


四阶抛物型方程
1.
In this paper,several new difference schemes for solving fourth order parabolic equation are developed by using dissipative term,and their orders of the local trumcation error and stability are discussed.
利用加耗散项的方法,提出解四阶抛物型方程的若干新的差分格式,研究它们的局部截断误差阶及稳定性。
2.
In this paper,we mainly consider the large time behavior of global solutions to the Cauchy problem of fourth order parabolic equation in one dimension space:with f(u) satisfyingHere are our main results:(i) Suppose the initial data satisfies .
本文主要考虑一维空间中四阶抛物型方程的Cauchy问题整体解u=u(x,t)的大时间行为。
3.
In this paper,we consider the large time behavior and the time-decay rate of global solutions to the Cauchy problem of fourth order parabolic equation in one dimension space: ■with f(u)satisfying f(u)∈C~1(■),|f(u)|≤C|u|~q,q>5/2.
本文考虑一维空间中四阶抛物型方程Cauchy问题■的整体解u=(x,t)的大时间渐近行为和时间衰减速率,其中
补充资料:抛物型偏微分方程
| 抛物型偏微分方程 parabolic type,partial differential equation of 偏微分方程的一类。最典型的是热传导方程 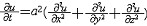 (a>0) (1)基本解是点热源的影响函数。若在t=0时在(ξ,η,ζ)处给定单位点热源,即u0(x0,y0,z0,0)=δ(ξ,η,ζ)(δ为狄拉克函数),则当t>0时便引起在R3的温度分布,这就是基本解。用傅里叶变换可得到它的表达式 (a>0) (1)基本解是点热源的影响函数。若在t=0时在(ξ,η,ζ)处给定单位点热源,即u0(x0,y0,z0,0)=δ(ξ,η,ζ)(δ为狄拉克函数),则当t>0时便引起在R3的温度分布,这就是基本解。用傅里叶变换可得到它的表达式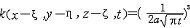 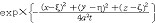 热传导方程初值问题的解可用基本解叠加而成,即 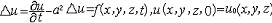 的解为 的解为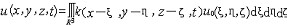 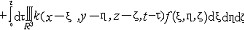 极值原理:一个内部有热源的传导过程,它的最低温度一定在边界上或初始时刻达到。更强的结论是 :如果t=T时在Ω内某一点达到最低温度 ,则在这个时刻以前(t<T时)u≡常数 ;又:若最低温度在t=T时边界¶Ω上某点P达到,则在这点上 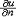 |P,Τ<0(n为外法线方向)。 |P,Τ<0(n为外法线方向)。 |
说明:补充资料仅用于学习参考,请勿用于其它任何用途。
参考词条