1) integral equation


积分方程式
2) integral equation


积分方程
1.
Computation of array induction logging response using integral equations;


利用积分方程计算阵列感应测井响应
2.
Multi-constraint smooth method for solving fredholm integral equation of first kind;


第一类Fredholm积分方程的多重约束光滑化方法
3.
Elimination of the singularity of integrands in the integral equation of harmonic electromagnetic field;
交变电磁场积分方程被积函数奇异性的消除
3) Integral equations


积分方程
1.
Study of live line measurement of parameters of transmission lines with mutual inductance based on integral equations;
基于积分方程的互感线路参数带电测量研究
2.
The application of fixed point theoretics to a kind of integral equations;


不动点理论在解一类积分方程中的应用
3.
But the abailable first or the second kind of integral equations are illposed, so that the regularization methods are used.
积分方程方法是求解波动逆问题的一种新的方法 ,它利用积分算子有效地将散射物边界数据遇射到远场或者近场测试的数据上 ,在已知散射物的初始物形和一些特征时 ,能给出较好的重构效果 ;但是 ,所得的第一类和第二类积分方程是不适定的 ,这样就需要用到正则化方法。
4) way of integration


积分方式
1.
By analyzing the experimental conditions and ways to establish the working curves,the experimental researches were done on the way of integration,which influences the exact value of threshold,and the correlation formula on different oil analysis results by MOA was given out.
通过分析MOA实验条件和工作曲线的建立方法,选取影响界限值确定的实验条件中的积分方式进行实验研究,建立了MOA检测不同油品的结果之间的相关表达式。
5) series equation way of integral format


级数形式积分方程法
6) integral form of the transport equation


输运方程的积分形式
补充资料:积分方程
| 积分方程 integral equation 积分号下含未知函数的方程。如果未知函数以线性形式出现于方程中,就叫线性积分方程 ,否则就称为非线性积分方程 。在积分方程正式出现之前 ,有的数学分支中的问题,事实上有了积分方程。如1782年,P.-S.拉普拉斯考虑过 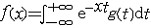 它是g(t)的积分方程 。在1823年S.D.泊松得到它的解为 它是g(t)的积分方程 。在1823年S.D.泊松得到它的解为 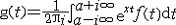 第一个自觉地直接应用并解出积分方程的人是N.H.阿贝尔 。他研究在地球引力场中的一个质点下落轨迹问题时提出的方程 ,是最早的积分方程: 第一个自觉地直接应用并解出积分方程的人是N.H.阿贝尔 。他研究在地球引力场中的一个质点下落轨迹问题时提出的方程 ,是最早的积分方程: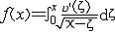 。他得到了解 。他得到了解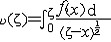 。 。积分方程一词是1888年P.du.B.雷蒙德提出的。1899年瑞典数学家E.I.弗雷德霍姆和意大利V.沃尔泰拉同时开创了线性积分方程方向,使积分方程理论逐步发展成一个数学分支。分别称以下的积分方程 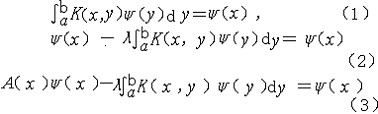 为第一类、二类、三类弗雷德霍姆积分方程。其中K(x,y )是在区域a≤x,y≤b上的连续函数,称为积分方程的核。 A(x),ψ(x)在a≤x≤b上连续,ψ(x)是未知函数 ,λ为参数。(1)与(2)都是(3)的特例,但(1)与(2)有本质的区别。 为第一类、二类、三类弗雷德霍姆积分方程。其中K(x,y )是在区域a≤x,y≤b上的连续函数,称为积分方程的核。 A(x),ψ(x)在a≤x≤b上连续,ψ(x)是未知函数 ,λ为参数。(1)与(2)都是(3)的特例,但(1)与(2)有本质的区别。沃尔泰拉也研究了三类方程,在(1)~(3)中将上限b换为x便是。如 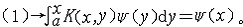 其他两个类似。但本质大不相同。 其他两个类似。但本质大不相同。D.希尔伯特和E.施密特对第二类弗雷德霍姆方程做了重要的工作,特别是关于对称核积分方程的特征值的存在性以及关于特征函数的展开,得到希尔伯特-施密特定理。 由于科学技术的需要 ,开 展了非线性积分方程的研究,发展很快。 |
说明:补充资料仅用于学习参考,请勿用于其它任何用途。
参考词条