2) Nonsymetric Linear Equations


线性非对称方程组
3) nonsymmetric linear systems


非对称线性方程组
1.
The Lanczos process is susceptible to possible breakdown and numerical instabilities of nonsymmetric linear systems.
在利用QMR方法求解非对称线性方程组(尤其是病态方程组)的Lanczos过程中通常会发生算法中断或数值不稳定的情况。
2.
To overcome this problem and improve the convergence,the augmented Krylov subspace technique is used by adding a few approximate eigenvectors associated to the eigenvalues that are close to zero and the deflated QMR method is given for nonsymmetric linear systems.
在利用QMR方法求解非对称线性方程组的Lanczos过程中通常会发生算法中断或数值不稳定的情况。
4) non-linear symmetrical equation sets


非线性对称方程组
1.
This paper mainly discusses the non-zero solution of non-linear symmetrical equation sets of S1=S2…=Sλ_1 = Sλ+1=…=Sn= Sn +1,(1≤λ≤n),(Sk= x1k+x2k+…xnk,k =0,1,2,…) and points out the structure of the solution sets.
主要讨论了非线性对称方程组S1=S2=…S2=…=Sλ-1=Sλ+1=…=Sn=Sn+1(1≤λ≤n),(Sk=x1k+x2k+…+xnk,k=0,1,2,…)的非零解,并指出其解集的结构。
6) unsymmetric linear system


非对称线性代数方程组
1.
An algorithm is proposed of the preconditioned generalized conjugate residual method for solving unsymmetric linear systems on a vector multiprocessor, when A is a five, seven or nine-diagonal matrix.
对五、七和九对角矩阵在并行一向量处理机上建立了解非对称线性代数方程组的PGCR算法,并分析了它的收敛性。
补充资料:线性方程组
| 线性方程组 linear equations,system of 各个方程关于未知量均为一次的方程组。对线性方程组的研究,中国比欧洲至少早1500年,记载在公元初《九章算术》方程章中。n个未知量m个方程的线性方程组的一般形式为 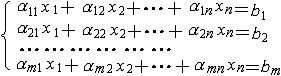 xj表未知量,aij称系数,bi称常数项。 xj表未知量,aij称系数,bi称常数项。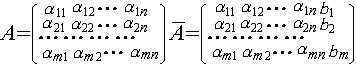 称为系数矩阵和增广矩阵。若x1=c1,x2=c2,…,xn=cn代入所给方程各式均成立,则称(c1,c2,…,cn)为一个解。若c1,c2,…,cn不全为0,则称(c1,c2,…,cn)为非零解。若常数项均为0,则称为齐次线性方程组,它总有零解(0,0,…,0)。两个方程组,若它们的未知量个数相同且解集相等,则称为同解方程组。线性方程组主要讨论的问题是:①一个方程组何时有解。②有解方程组解的个数。③对有解方程组求解,并决定解的结构。这几个问题均得到完满解决:所给方程组有解 称为系数矩阵和增广矩阵。若x1=c1,x2=c2,…,xn=cn代入所给方程各式均成立,则称(c1,c2,…,cn)为一个解。若c1,c2,…,cn不全为0,则称(c1,c2,…,cn)为非零解。若常数项均为0,则称为齐次线性方程组,它总有零解(0,0,…,0)。两个方程组,若它们的未知量个数相同且解集相等,则称为同解方程组。线性方程组主要讨论的问题是:①一个方程组何时有解。②有解方程组解的个数。③对有解方程组求解,并决定解的结构。这几个问题均得到完满解决:所给方程组有解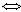 秩(A)=秩 秩(A)=秩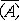 ;若秩(A)=秩 ;若秩(A)=秩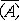 =r,则r=n时,有唯一解;r<n时,有无穷多解;可用消元法求解。克莱姆法则(见行列式)给出了一类特殊线性方程组解的公式。n个未知量的任一齐次方程组的解集均构成n维空间的一个子空间。 =r,则r=n时,有唯一解;r<n时,有无穷多解;可用消元法求解。克莱姆法则(见行列式)给出了一类特殊线性方程组解的公式。n个未知量的任一齐次方程组的解集均构成n维空间的一个子空间。线性方程组有广泛应用,熟知的线性规划问题即讨论对解有一定约束条件的线性方程组问题。 |
说明:补充资料仅用于学习参考,请勿用于其它任何用途。
参考词条
 专业词汇(按中图法分类)
专业词汇(按中图法分类)