1) Lipschitz space associated with parabola


抛物型Lipschitz空间
2) BMO spaces associated withparabola


抛物型BMO空间
3) parabolic space


抛物空间
4) Lipschitz space


Lipschitz空间
1.
And the function multipliers between Lipschitz spaces or from Lipschitz space to Bloch space are characterized.
在Cn中单位球上根据p,q的不同范围给出了Bloch型空间βp和βq之间函数乘子的一种新的刻划,并刻划了Lipschitz空间之间以及Lipschitz空间到Bloch空间的函数乘子,拓广了Bloch空间的乘子理论。
2.
In this paper, we discuss non-commutatitive Lipschitz spaces, non-commutatitive Lipschitz algebras and derivations in a Banach algebra.
本文研究了由矩阵值Lipschitz-α映射构成的非交换Lipschitz空间和非交换Lipschitz代数,讨论了复Banach代数上内导子,而且给出了解析函数的Lipschitz性质。
3.
Furthermore, we proved the multilinear commutator of multiplier operator T~(?), which is generated by multiplier operator and functions in Lipschitz space, is bounded on Lipschitz space, Hardy space and is weak bounded in Hardy space .
然后,讨论了乘子算子与Lipschitz函数生成的多线性交换子T~(?)在Lipschitz空间,Hardy空间上的强有界及弱有界性。
5) Lipschitz martingale space


Lipschitz鞅空间
补充资料:抛物型偏微分方程
| 抛物型偏微分方程 parabolic type,partial differential equation of 偏微分方程的一类。最典型的是热传导方程 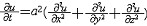 (a>0) (1)基本解是点热源的影响函数。若在t=0时在(ξ,η,ζ)处给定单位点热源,即u0(x0,y0,z0,0)=δ(ξ,η,ζ)(δ为狄拉克函数),则当t>0时便引起在R3的温度分布,这就是基本解。用傅里叶变换可得到它的表达式 (a>0) (1)基本解是点热源的影响函数。若在t=0时在(ξ,η,ζ)处给定单位点热源,即u0(x0,y0,z0,0)=δ(ξ,η,ζ)(δ为狄拉克函数),则当t>0时便引起在R3的温度分布,这就是基本解。用傅里叶变换可得到它的表达式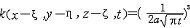 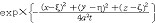 热传导方程初值问题的解可用基本解叠加而成,即 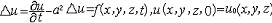 的解为 的解为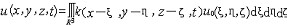 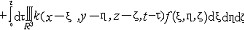 极值原理:一个内部有热源的传导过程,它的最低温度一定在边界上或初始时刻达到。更强的结论是 :如果t=T时在Ω内某一点达到最低温度 ,则在这个时刻以前(t<T时)u≡常数 ;又:若最低温度在t=T时边界¶Ω上某点P达到,则在这点上 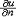 |P,Τ<0(n为外法线方向)。 |P,Τ<0(n为外法线方向)。 |
说明:补充资料仅用于学习参考,请勿用于其它任何用途。
参考词条