1) third order semilinear pseudoparabolic boundary value problems


三阶伪抛物型方程
2) pseudoparabolic equation


伪抛物型方程
1.
This paper deals with a class of inverse problems of determining the unknown source term for the pseudoparabolic equation.
讨论了一类伪抛物型方程未知源项的存在性和唯一性问题。
2.
This paper deals with a class of inverse problems to determine the constant coefficient for the pseudoparabolic equation with special initial condition.
文章讨论了一类具有特殊初值条件的伪抛物型方程确定常数系数的反问题。
3.
Combining Riemann′s method with the fixed point theory effectively,we study a class of backwards heat flow problems of one-dimensional nonlinear pseudoparabolic equations,and obtain the existence and uniqueness of the solution of the inverse problem.
将Riemann函数方法与不动点理论有效地结合起来,研究了一类一维非线性伪抛物型方程的后向热流问题,得出了反问题解的存在唯一性结论。
3) pseudo-parabolic equation


伪抛物型方程
1.
A weak solution to the viscous Laplacian evolution equation with nonlinear source(a pseudo-parabolic equation) is proved to be in existence by using the time-discrete method.
用时间离散化方法证明了一个粘性非线性源的p-Lap lace发展方程(此方程为伪抛物型方程)初边值问题弱解的存在性。
2.
The first type studied in this paper is pseudo-parabolic equation.


研究的第一类发展型方程——伪抛物型方程具有广泛的应用背景,特别在非传统密码体制——热流密码体制中也有应用模型。
4) pseudoparbolic equation system


伪抛物型方程组
5) Pseudoparabolic Complex Equation


伪抛物型复方程
6) four order parabolic equations


四阶抛物型方程
1.
A two-level explicit finite difference scheme of high accuracy for solving four order parabolic equations is presented,the stability condition of the presented scheme is r=τ/h4≤264/3601 and the truncation order iso(τ2+h8).
给出了一个求解四阶抛物型方程高精度两层显式差分格式,证明了其截断误差为O(τ2+h8),稳定性条件为r=τ/h4≤264/3601。
2.
A ten-point two-level explicit finite difference scheme to solve four order parabolic equations is presented,and it is demonstrated that the stability condition of the presented scheme is ο(τ2+h4) and the truncation order is r=τh4≤79384.
给出了一个求解四阶抛物型方程的两层十点显式差分格式,证明了其截断误差为ο(τ2+h4),稳定性条件为r=hτ4≤37894。
补充资料:抛物型偏微分方程
| 抛物型偏微分方程 parabolic type,partial differential equation of 偏微分方程的一类。最典型的是热传导方程 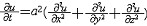 (a>0) (1)基本解是点热源的影响函数。若在t=0时在(ξ,η,ζ)处给定单位点热源,即u0(x0,y0,z0,0)=δ(ξ,η,ζ)(δ为狄拉克函数),则当t>0时便引起在R3的温度分布,这就是基本解。用傅里叶变换可得到它的表达式 (a>0) (1)基本解是点热源的影响函数。若在t=0时在(ξ,η,ζ)处给定单位点热源,即u0(x0,y0,z0,0)=δ(ξ,η,ζ)(δ为狄拉克函数),则当t>0时便引起在R3的温度分布,这就是基本解。用傅里叶变换可得到它的表达式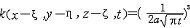 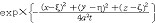 热传导方程初值问题的解可用基本解叠加而成,即 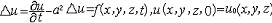 的解为 的解为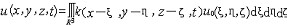 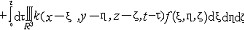 极值原理:一个内部有热源的传导过程,它的最低温度一定在边界上或初始时刻达到。更强的结论是 :如果t=T时在Ω内某一点达到最低温度 ,则在这个时刻以前(t<T时)u≡常数 ;又:若最低温度在t=T时边界¶Ω上某点P达到,则在这点上 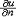 |P,Τ<0(n为外法线方向)。 |P,Τ<0(n为外法线方向)。 |
说明:补充资料仅用于学习参考,请勿用于其它任何用途。
参考词条