1) Singular parabolic equation


奇异抛物型方程
3) degenerate parabolic equation


退化奇异抛物方程
1.
Blow-up for degenerate parabolic equation with nonlocal source and absorption;


带非局部源和吸收项的退化奇异抛物方程的爆破
4) quasilinear singular parabolic equation


拟线性奇异抛物方程
1.
The present article employs the non-standard Galerkin finite element method to discuss the weighted L2 model of the strange parabolic equation and |||·||| model error estimate,and analyses the quasilinear singular parabolic equation,getting the error estimates of the weighted L2 model |||·||| model.
模误差估计;对拟线性奇异抛物方程进行了分析并得到了加权L2模和。
补充资料:抛物型偏微分方程
| 抛物型偏微分方程 parabolic type,partial differential equation of 偏微分方程的一类。最典型的是热传导方程 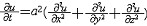 (a>0) (1)基本解是点热源的影响函数。若在t=0时在(ξ,η,ζ)处给定单位点热源,即u0(x0,y0,z0,0)=δ(ξ,η,ζ)(δ为狄拉克函数),则当t>0时便引起在R3的温度分布,这就是基本解。用傅里叶变换可得到它的表达式 (a>0) (1)基本解是点热源的影响函数。若在t=0时在(ξ,η,ζ)处给定单位点热源,即u0(x0,y0,z0,0)=δ(ξ,η,ζ)(δ为狄拉克函数),则当t>0时便引起在R3的温度分布,这就是基本解。用傅里叶变换可得到它的表达式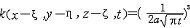 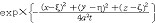 热传导方程初值问题的解可用基本解叠加而成,即 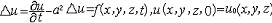 的解为 的解为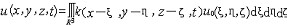 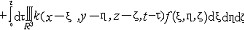 极值原理:一个内部有热源的传导过程,它的最低温度一定在边界上或初始时刻达到。更强的结论是 :如果t=T时在Ω内某一点达到最低温度 ,则在这个时刻以前(t<T时)u≡常数 ;又:若最低温度在t=T时边界¶Ω上某点P达到,则在这点上 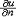 |P,Τ<0(n为外法线方向)。 |P,Τ<0(n为外法线方向)。 |
说明:补充资料仅用于学习参考,请勿用于其它任何用途。
参考词条