1) Linear Quasi-hyperbolic Integro-differential Equation


伪双曲型积分微分方程
1.
(1) Linear Quasi-parabolic Integro-differential Equation(2)Linear Quasi-hyperbolic Integro-differential EquationWe obtain Lp-optimal and W1,p- optimal estimats under the certain condition(2 ≤ p < ∞).
本文的第一、二章分别考虑(1)伪抛物型积分微分方程的初边值问题(2)伪双曲型积分微分方程的初边值问题的有限元超收敛结果。
2) Hyperbolic Integrodifferential Equation


双曲型积分微分方程
1.
Finite element method for semilinear pseudo hyperbolic integrodifferential equations mixed initial boundary value problem is studied.
研究了半线性拟双曲型积分微分方程的一类混合初边值问题的有限元方法,引入Ritz-Voltera投影方法,得到了半离散有限元格式的最优阶误差估计。
3) Linear Quasi-parabolic Integro-differential Equation


伪抛物型积分微分方程
1.
(1) Linear Quasi-parabolic Integro-differential Equation(2)Linear Quasi-hyperbolic Integro-differential EquationWe obtain Lp-optimal and W1,p- optimal estimats under the certain condition(2 ≤ p < ∞).
本文的第一、二章分别考虑(1)伪抛物型积分微分方程的初边值问题(2)伪双曲型积分微分方程的初边值问题的有限元超收敛结果。
5) hyperbolic differential equation


双曲型微分方程
1.
Some necessary and sufficient conditions for the oscillation of solutions of delay hyperbolic differential equations are obtained.
建立了一类时滞双曲型微分方程解的振动充要条件,揭示了这类双曲方程与相应泛函微分方程解的振动的等价性。
2.
By using a generalized Riccati transformation, some sufficient conditions are established for the oscillation of solutions of delay hyperbolic differential equations of the form ~2 t~2u(x,t) =a(t)Δu(x,t)+sk=1a_k(t)Δ u(x,t-ρ_k)-mj=1q_j(x,t)u(x,t-σ_j), where (x,t)∈Ω×[0,∞)≡G, Ω is a bounded domain in R~N with a piecewise smooth boundary Ω and Δ is the Laplacian in Euclidean N-space R~N.
利用广义Riccati变换 ,建立了下列时滞双曲型微分方程 2 t2 u(x ,t) =a(t)Δu(x ,t) + sk =1ak(t)Δu(x ,t- ρk) - mj =1qj(x,t)u(x,t-σj)解的振动的若干充分条件 ,其中 (x ,t)∈Ω× [0 ,∞ )≡G ,Ω是RN中具有逐片光滑边界 Ω的有界区域 ,Δu(x ,t) = Nr=1 2 u(x ,t) x2r。
3.
In this paper,by using the characteristic equation,some forced oscillation of certain delay hyperbolic differential equations are obtained.
借助其特征方程 ,获得了一类时滞双曲型微分方程解的强迫振动的若干充分条
6) hyperbolic equation


双曲型微分方程
1.
This paper deals with the Cauchy problem for a hyperbolic equation of second order by transforming the problem into a system of integral equations,thus proving that the problem has differentiable solution under some conditions by using the iteration method.
研究了二阶双曲型微分方程沿着一组特征线的柯西问题 ,处理这个问题的方法是通过引入辅助函数 ,转化为求解积分方程组 ,并利用迭代法 ,证明了在一定条件下这个二阶双曲型微分方程的柯西问题有
2.
Deals with the Cauchy problem for a hyperbolic equation of second order v xx -h(x,y)k(y)v yy +a(x,y)v x+b(x,y)v y+c(x,y)v+f(x,y)=0.
研究了一类二阶双曲型微分方程 vxx-h( x,y) k( y) vyy+ a( x,y) vx+ b( x,y) vy+ c( x,y) v+ f ( x,y) =0的柯西问题解的存在性 。
补充资料:双曲型偏微分方程
| 双曲型偏微分方程 hyperbolic type,partial differential equation of 描述振动或波动现象的偏微分方程。它的一个典型特例是波动方程 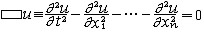 n=1时的波动方程 n=1时的波动方程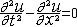 可用来描述弦的微小横振动,称为弦振动方程。这是最早得到系统研究的一个偏微分方程。 可用来描述弦的微小横振动,称为弦振动方程。这是最早得到系统研究的一个偏微分方程。 |
说明:补充资料仅用于学习参考,请勿用于其它任何用途。
参考词条