2) neutral parabolic equation


中立抛物型方程
1.
Oscillation of solutions of a class of quasilinear impulsive neutral parabolic equations was investigated and several sufficient criteria for oscillation of such systems with two boundary conditions were established in the present paper.
研究了一类拟线性脉冲中立抛物型方程解的振动性,在两类边界条件下,建立了方程解振动的几个充分性准则。
3) neutral type delay parabolic differential equation


中立型时滞抛物型方程
4) parabolic equations of neutral type


中立型抛物方程组
1.
In this paper,we investigate the oscillation criteria for a class of systems of nonlinear parabolic equations of neutral type with the technique of spatial average.
本文讨论了一类拟线性多滞量中立型抛物方程组解的振动性质,利用空间平均法,获得了判别其解振动的充分条件,表明了时滞量对方程振动性的影响。
5) neutral delay parabolic equation


中立型时滞抛物方程
1.
Oscillation of solutions for a class of impulsive neutral delay parabolic equation;


一类脉冲中立型时滞抛物方程的振动性
6) parabolic neutral type


抛物中立型
补充资料:抛物型偏微分方程
| 抛物型偏微分方程 parabolic type,partial differential equation of 偏微分方程的一类。最典型的是热传导方程 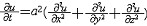 (a>0) (1)基本解是点热源的影响函数。若在t=0时在(ξ,η,ζ)处给定单位点热源,即u0(x0,y0,z0,0)=δ(ξ,η,ζ)(δ为狄拉克函数),则当t>0时便引起在R3的温度分布,这就是基本解。用傅里叶变换可得到它的表达式 (a>0) (1)基本解是点热源的影响函数。若在t=0时在(ξ,η,ζ)处给定单位点热源,即u0(x0,y0,z0,0)=δ(ξ,η,ζ)(δ为狄拉克函数),则当t>0时便引起在R3的温度分布,这就是基本解。用傅里叶变换可得到它的表达式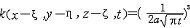 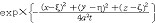 热传导方程初值问题的解可用基本解叠加而成,即 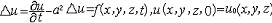 的解为 的解为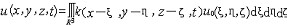 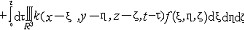 极值原理:一个内部有热源的传导过程,它的最低温度一定在边界上或初始时刻达到。更强的结论是 :如果t=T时在Ω内某一点达到最低温度 ,则在这个时刻以前(t<T时)u≡常数 ;又:若最低温度在t=T时边界¶Ω上某点P达到,则在这点上 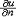 |P,Τ<0(n为外法线方向)。 |P,Τ<0(n为外法线方向)。 |
说明:补充资料仅用于学习参考,请勿用于其它任何用途。
参考词条