1) extension of a function
函数的扩张
2) extension of continuous function
连续函数的扩张
3) extend functions
μ-扩张函数
4) extensible shift function
可扩张位移函数
5) augmented Lagrangian function
扩张性拉氏函数
1.
Presents again a new formulation of Lagrange s equations by using an augmented Lagrangian function with the second order non linear nonholonomic constraint equations.
采用带有二阶非线性非完整约束方程的扩张性拉氏函数 ,建立起了拉格朗日方程的又一新公式 ,该新公式较为规则 ,它提供了一种完整约束系统和非完整约束系统两者统一的变分方法。
2.
Presents a new formulation of Lagrange s equations by using an augmented Lagrangian function with the first order non linear nonholonomic constraint equations, and offers a unified variational approach to both holonomic and nonholonomic constraints.
并指出了一个“漂亮”的扩张性拉氏函数的拉格朗日方程的错误。
6) left and right spreads
左(右)扩张函数
补充资料：连续函数
| 连续函数 continuous function 函数y＝f（x）当自变量x的变化很小时，所引起的因变量y的变化也很小。例如，气温随时间变化，只要时间变化很小，气温的变化也是很小的；又如，自由落体的位移随时间变化，只要时间变化足够短，位移的变化也是很小的，对于这种现象，我们说因变量关于自变量是连续变化的，可用极限给出严格描述：设函数y＝f（x）在x0点附近有定义，如果 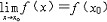 ，则称函数f在x0点连续。如果定义在区间I上的函数在每一点x∈I都连续，则说f在I上连续，此时，它在直角坐标系中的图像是一条没有断裂的连续曲线。 |
说明：补充资料仅用于学习参考，请勿用于其它任何用途。
参考词条