1) complete and compact metric spaces


完备度量空间和紧度量空间
1.
Here, we provide two new fixed point theorems in complete and compact metric spaces into which the concept of a w-distance was introduced.
在完备度量空间和紧度量空间上引入了w-距离,从而得到了两个新的不动点定理。
2) complete metric space


完备度量空间
1.
Fixed points on complete metric spaces;


完备度量空间中的不动点(英文)
2.
Uses the property of complete metric space and lemma [1.


利用完备度量空间的性质和引理[1。
3.
Using the property of complete metric space and related lemmas 1 and 2,the existence of common fixed point of a couple of fuzzy contractive mappings with inequality conditions and the cut set being nonempty closed bounded subsets of complete metric space X,is studied;and several theorems on the existence of common fixed point are given.
利用完备度量空间的性质和引理1、2,研究了在完备度量空间X中一对压缩型模糊映象当其截集是X中非空有界闭集时,该对压缩型模糊映象的公共不动点的存在性问题,推广了Vija-yaraju P和Marudai M论文的结论。
3) complete metric spaces


完备度量空间
1.
A new fixed point theorem in complete metric spaces for four mappings;


完备度量空间中四个映象的一个新的不动点定理
2.
Fisher B proved the following fixed point theorem:Let (X,d) and (Y,ρ) be complete metric spaces,let T be a continuous mapping of X into Y and let S be a mapping of Y into X satisfying the inequalities d (STx,STx′)≤C max { d (x,x′), d (x,STx), d (x′,STx′),ρ(Tx,Tx′)}ρ(TSy,TSy′)≤C max {ρ(y,y′),ρ(y,TSy),ρ(y′,TSy′),d(Sy,Sy′)} for all x,x′ in X and in Y,where 0≤C<1.
该文对此定理作一推广,从而得到了完备度量空间与紧度量空间上2 个新的不动点定理。
3.
By using the definition for compatible self-mappings in metric spaces,the existence of common fixed point for Φ expansive compatible mappings in complete metric spaces is considered.
利用度量空间中自映射对相容的定义,讨论了完备度量空间中Φ扩张相容映射公共不动点的存在性,推广和改进了张石生、谷峰等人一些相关的结果。
4) convex metric space


完备凸度量空间
1.
On the convergence of the Ishikawa iterates to a common fixed point of two mappings in complete convex metric spaces;
完备凸度量空间中两个映射的公共不动点的Ishikawa迭代强收敛定理
2.
The theorems on Ishikawa ierates strongly converging to a common fixed point for two mappings in complete convex metric spaces;
完备凸度量空间中Ishikawa迭代序列强收敛到两个映射的公共不动点定理
5) Complete Fuzzy metric spaces


完备Fuzzy度量空间
补充资料:度量空间
| 度量空间 metric space 具有度量的抽象空间,设X是一个集合,若有定义在X×X上的非负实值函数d,满足①d(x,y)≥0,d(x,y)=0 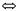 x=y; ②d(x,y)=d(y,x);③d(x,z)≤d(x,y)+d(y,z),则称(X,d)是度量空间,d称为距离或度量。这是最接近于欧几里得空间的抽象空间。利用度量可很自然地将欧几里得空间上点的邻域、开集、闭集,收敛序列以及连续映射等概念推广到一般度量空间,也能将一致连续的概念推广到度量空间。由于19世纪末集合论产生后,实变函数及泛函分析的发展,需要规定函数间的距离,因而抽象出度量、度量空间的概念,其创始人是M.R.弗雷歇。常见的度量空间有: x=y; ②d(x,y)=d(y,x);③d(x,z)≤d(x,y)+d(y,z),则称(X,d)是度量空间,d称为距离或度量。这是最接近于欧几里得空间的抽象空间。利用度量可很自然地将欧几里得空间上点的邻域、开集、闭集,收敛序列以及连续映射等概念推广到一般度量空间,也能将一致连续的概念推广到度量空间。由于19世纪末集合论产生后,实变函数及泛函分析的发展,需要规定函数间的距离,因而抽象出度量、度量空间的概念,其创始人是M.R.弗雷歇。常见的度量空间有:n维欧几里得空间(Rn,d):Rn={(x1,…,xn)|xi∈R,i=1,2,…,n },d(x,y)= 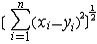 ,其中x=(x1,x2,…, xn),y=(y1,y2,…,yn)。 ,其中x=(x1,x2,…, xn),y=(y1,y2,…,yn)。希尔 伯特空 间(l2;d):l2={(x1,x2,…,xn…) 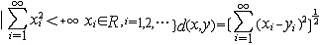 , 其中x =( x1,x2 ,…),y=(y1,y2,…)∈l2。 , 其中x =( x1,x2 ,…),y=(y1,y2,…)∈l2。函数空间(ρ[0,1],d):C[0,1]={f:f为[0,1]上的实值连续函数},对任意f,g∈C[0,1],d(f,g)=max{|f(x)-g(x)|}。 x∈[0,1] 对度量空间(X,d)可引进拓扑结构,即以包含开球B(x,r)={y∈X|d( x,y)<r }的集为邻域定义拓扑,称为d所诱导的拓扑。 |
说明:补充资料仅用于学习参考,请勿用于其它任何用途。
参考词条