1) Higher-order parabolic system


高阶抛物型方程组
2) parabolic equation of higher order


高阶抛物型方程
1.
A three-layer explicit difference scheme is proposed for solving the parabolic equation of higher order [SX(] u[] t[SX)]=(-1) m+1 [SX(] 2m u[] t 2m [SX)] (where m is a positive integer).
对高阶抛物型方程提出一个三层显式差分格式,其局部截断误差阶是O(τ2+h4)。
2.
For solving the parabolic equation of higher order [SX(] u[] t[SX)]=(-1) m+1 [SX(] 2m u[] x 2m [SX)] (where m is a positive integer), a family of three-layered implicit difference schemes containing biparameters are constructed.
对高阶抛物型方程t=(-1)m+1x2m(m为正整数),构造一族含双参数的三层隐式差分格式·在特殊情况下,当参数α=21,β=0时得到一个双层格式·这些格式的截断误差阶均为O((Δt)2+(Δx)4)。
3) high order parabolic equation


高阶抛物型方程
1.
For Solving high order parabolic equation = (?1)m+1 ?t (where m is positive inerger), the author advances a ?x2m two-layer implicit difference scheme with order of local truncation error o(τ + h4) , and when m = 1, 2, 3 , the scheme is proved to 2 be absolutely stable.
本文构造出解高阶抛物型方程=(?1)m+12?t?x2m(m为正整数)的局部截断误差阶为o(τ+h4)的两层隐式差分格式,并证明了当m=1,2,3是它是绝对稳定的。
4) second-order parabolic system


二阶抛物型方程组
1.
In Chapter One,a new method of approximating the solution of second-order parabolic system using reproducing kernel function is devised.
第一章研究了一类二阶抛物型方程组的一种新数值方法-再生核函数法。
6) fourth order parabolic equations


四阶抛物方程组
1.
In this paper, we mainly consider the global existence of the solutions, large time behavior and the L~1-time decay of the fourth order parabolic equations.
在这篇文章中我们主要考虑如下一维空间中的四阶抛物方程组柯西问题整体解的存在性,大时间行为和L~1时间衰减速率。
补充资料:抛物型偏微分方程
| 抛物型偏微分方程 parabolic type,partial differential equation of 偏微分方程的一类。最典型的是热传导方程 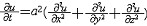 (a>0) (1)基本解是点热源的影响函数。若在t=0时在(ξ,η,ζ)处给定单位点热源,即u0(x0,y0,z0,0)=δ(ξ,η,ζ)(δ为狄拉克函数),则当t>0时便引起在R3的温度分布,这就是基本解。用傅里叶变换可得到它的表达式 (a>0) (1)基本解是点热源的影响函数。若在t=0时在(ξ,η,ζ)处给定单位点热源,即u0(x0,y0,z0,0)=δ(ξ,η,ζ)(δ为狄拉克函数),则当t>0时便引起在R3的温度分布,这就是基本解。用傅里叶变换可得到它的表达式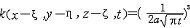 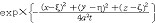 热传导方程初值问题的解可用基本解叠加而成,即 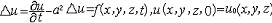 的解为 的解为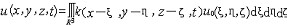 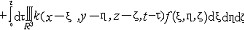 极值原理:一个内部有热源的传导过程,它的最低温度一定在边界上或初始时刻达到。更强的结论是 :如果t=T时在Ω内某一点达到最低温度 ,则在这个时刻以前(t<T时)u≡常数 ;又:若最低温度在t=T时边界¶Ω上某点P达到,则在这点上 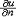 |P,Τ<0(n为外法线方向)。 |P,Τ<0(n为外法线方向)。 |
说明:补充资料仅用于学习参考,请勿用于其它任何用途。
参考词条