1) meromorphic close-to-convex


亚纯近于凸函数
2) meromorphic convex function


亚纯凸函数
1.
In this paper, some porperties of Hadamard products of the class σk(α) of meromorphic convex functions with negative coefficients are investigated with the use of some results in reference
利用文献的一些结果,研究了具有负系数的亚纯凸函数族σk(α)的Hadamard乘积的一些性质
3) close-to-convex function


近于凸函数
1.
Sharp results concerning coefficients,distortion,covering theorems for the close-to-convex functions with negative coefficients are investigated.
该文利用复分析中的一些初等方法,讨论了具有负系数的近于凸函数族精确的系数估计,偏差定理,闭包定理,极值点问题及在分数次微积分上的应用,得到了其准确的结果,从而推广了一些作者的相关结果。
2.
We introduce a extension of the class of close-to-convex functions to p-valent,obtain inclusion relation.
给出了P叶近于凸函数类的一个扩展。
3.
The integral operator Pσλf(z) was used to describe starlike function,convex function close-to-convex function and the new subclass of quasi convex function and to establish inclusion relations.
用积分算子Pσλf(z)刻划了星象函数,凸象函数,近于凸函数,拟凸函数的新子类,建立了包含关系。
4) close-to-convex functions


近于凸函数
1.
The radius of close-to-convex functions,starlike functions,convex functions were also considered.
利用Ruscheweyh导数算子引进了单位圆盘内解析函数的一个新子类,给出了函数属于函数类的两个充要条件,并考虑了近于凸函数、星象函数和凸函数半径。
5) meromorphic convex


亚纯凸象函数
6) meromorphic quasi-convex


亚纯拟凸函数
补充资料:亚纯函数
| 亚纯函数 meromorphic function 在区域D上有定义 ,且除去极点之外处处解析的函数。比如有理函数就是在扩充复平面上的亚纯函数,它是两个多项式的商 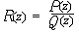 ,而Q(z)的零点是R(z)的极点 ,即R(z)有有限多个极点,∞点是R(z)的极点或可去奇点 。复平面上不是有理函数的亚纯函数称为超越亚纯函数。例如ctgz就是超越亚纯函数 ,它以kπ为全部极点 ,超越亚纯函数一定有无限多个极点。有理函数可以分为部分分式,即 
说明:补充资料仅用于学习参考,请勿用于其它任何用途。
参考词条
|
|
| ©2011 dictall.com | |