1) composite entire function


复合整函数
1.
Order and type of a class of composite entire function;


一类复合整函数的级与型
2) integrative function


整合函数
3) compound function


复合函数
1.
Analyzing an important elements—analyzing compound function of differential and integral calculating;
微积分运算中的重要基础——分解复合函数
2.
A footnote about derivation rule of compound function;


关于复合函数的求导法则所进行的一个注脚
3.
The absolute continuity of a compound function


关于一类复合函数的绝对连续性
4) functional composition


函数复合
1.
This paper deals with this problem by use of functional composition.


在本文中,我们用函数复合的方法实现两者之间的相互转化。
5) Composite function


复合函数
1.
Methods to judeg whether composite functions are even or odd;


复合函数奇偶性的判断方法
2.
A composite function involving the Smarandache function;


一个包含Smarandache函数的复合函数
3.
Improvement of composite function analytic property in advanced mathematics;


复合函数分析性质的改进
6) function of functions


复合函数
1.
A detailed discussion on the limit of function of functions is taken and six theorems are given in this paper.
本文对复合函数的极限做了细致的讨论 ,给出了 6个定
2.
Through the applications in the integration by substitution, finding the value of whole differential equation, differentiate and partial derivative or partial derivative of higher order for multivariate (one variable) function of functions, this paper discusses that the function of the invariance of differential form of first order should be not neglected in calculus.
通过在积分换元、微分方程求解、多(一)元复合函数求全微分、偏导数及高阶偏导数中的应用举例,论述了一阶微分的形式不变性在微积分学中的作用不应被忽略。
补充资料:整函数
| 整函数 integral function 在整个复平面上处处解析的函数。整函数总可以在原点 展开成泰勒级数:  ,它在全平面收敛,整函数以∞点为唯一的孤立奇点,它在∞点的罗朗展式与它在原点的泰勒展式有一样的形式。当∞点是整函数的可去奇点时,这个整函数只能是常数,这就是著名的刘维尔定理,通常表述为“有界整函数必为常数”。利用这一定理可以得到代数基本定理的简单证明。当∞点是整函数的n阶极点时,这个整函数是一个n次多项式 ,也就是它的泰勒展式(或罗朗展式)只有有限多项。当∞点是整函数的本性奇点时,这个整函数的泰勒展式一定有无限多项,这类整函数称为超越整函数。由代数基本定理知道n次多项式一定有n个零点(也就是根),它总可以分解为n个一次因式的积,对于超越整函数,它可能有无限多个零点 ,比如sinπz就以全体整数为其零点集,也有的超越整函数没有零点,如ez就处处不为零,一般来说,没有零点的超越整函数总可以表成eg(z)的形式,此处g(z)也是一个整函数,而有无限多个零点的超越整函数f(z)也有一个因子分解式 ;形如 ,它在全平面收敛,整函数以∞点为唯一的孤立奇点,它在∞点的罗朗展式与它在原点的泰勒展式有一样的形式。当∞点是整函数的可去奇点时,这个整函数只能是常数,这就是著名的刘维尔定理,通常表述为“有界整函数必为常数”。利用这一定理可以得到代数基本定理的简单证明。当∞点是整函数的n阶极点时,这个整函数是一个n次多项式 ,也就是它的泰勒展式(或罗朗展式)只有有限多项。当∞点是整函数的本性奇点时,这个整函数的泰勒展式一定有无限多项,这类整函数称为超越整函数。由代数基本定理知道n次多项式一定有n个零点(也就是根),它总可以分解为n个一次因式的积,对于超越整函数,它可能有无限多个零点 ,比如sinπz就以全体整数为其零点集,也有的超越整函数没有零点,如ez就处处不为零,一般来说,没有零点的超越整函数总可以表成eg(z)的形式,此处g(z)也是一个整函数,而有无限多个零点的超越整函数f(z)也有一个因子分解式 ;形如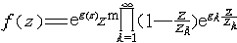 ,其中g(z)是整函数,0是m阶零点,zk是非零零点集,gk( ,其中g(z)是整函数,0是m阶零点,zk是非零零点集,gk( )是 )是 的多项式,这是魏尔斯托拉斯因子分解定理。超越整函数还有一个重要性质:若f(z)是超越整函数,则对任意复数A(包括A=∞),存在点列{zk },使zk 的多项式,这是魏尔斯托拉斯因子分解定理。超越整函数还有一个重要性质:若f(z)是超越整函数,则对任意复数A(包括A=∞),存在点列{zk },使zk  ∞(k ∞(k ∞)而有f(zk) ∞)而有f(zk) A。这一结果有一个更精确的发展:对超越整函数f(z),最多除去一个值(称为例外值)外,对所有其他的复数v值(v≠∞),f(z)-v都有无穷多个零点(毕卡定理)。 A。这一结果有一个更精确的发展:对超越整函数f(z),最多除去一个值(称为例外值)外,对所有其他的复数v值(v≠∞),f(z)-v都有无穷多个零点(毕卡定理)。 |
说明:补充资料仅用于学习参考,请勿用于其它任何用途。
参考词条