1) Irrational number


无理数
1.
This paper attempts to popularize the two conclusions in elementary mathematics:the deduction of "2 is one of the irrational numbers" and "the thermo of internal bisector for triangle".
推广了初等数学中“1/2是无理数”和“三角形内角平分线定理”两个推论,并给出了初等数学方法证明。
2.
This article,according to the power series expansion and the Leibniz theorem,had proven,using the reduction to absurdity,when n is the non-vanishing integer,sin(l/n) is an irrational number.
根据sinx的幂级数展开式和莱布尼茨定理,利用反证法证明了当n为非零整数时,sin(1/n)为无理数。
3.
A Taylor formula with integral form surplus term is deduced,by means of which e is simply proved to be an irrational number.
推导一种带有积分形式余项的Taylor公式,并用这个公式比较简单地证明e是无理数。
2) irrational
[英][ɪ'ræʃənl] [美][ɪ'ræʃənḷ]


无理数
1.
Sum of square roots of prime numbers is irrational;


素数的平方根之和是一个无理数
2.
The generalized Fibonacci sequence can prove that square roots of integer is either integer or irrational.
文章引入一类广义斐波那契数列,给出其收敛的充分必要条件,并利用该类广义斐波那契数列证明了任何自然数的算术平方根或是自然数或是无理数。
3.
In the paper,I research into the relation between the recurring continued fraction and the quadratic irrational Firstly I prove any recurring continued faction is all the quadratic irrational,and then offer the general method changing the recurring continued fraction into the quadratic irrationa
研究循环连分数与二次无理数关系问题 ,首先证明了任何循环连分数皆为二次无理数 ,并给出化循环连分数为二次无理数的一般方
3) irrational numbers


无理数
1.
DES and AES method for data encryption are discussed in this paper,and the analysis of security is also given,then an improved scheme based on irrational numbers is proposed.
首先介绍数据加密标准(Data Encryption Standard,DES)和高级加密标准(Advanced Encryption Standard,AES),并对其安全性进行分析,然后提出基于无理数的DES加密方案。
4) irrational function


无理函数
1.
The integration by second substitution is an important method of calculating indefinite integral,it has a certain application,it usually applies to calculate some integrals of irrational function.
第二换元积分法是求函数不定积分的一种重要方法,具有一定的适用范围,对某些无理函数的积分的求解通常使用该方法。
2.
It is difficult to find the solutions to some complex irrational functions and even worse, there is no solutions to them.
有些比较复杂的无理函数的积分,用传统的方法求解有困难,甚至无法积分出来,而用组合积分法可以巧妙地解决无法积分的问题。
3.
By describing states Euler s transformation of indefinite integral of irrational function,the author analyses what is Euler s transformation through Choosing Q(t) ,and reveals the fundamentals of Euler s transformation.
阐明了求无理函数不定积分的欧拉变换 ,通过选取Q(t)的方法分析了欧拉变换的来龙去脉 ,揭示出欧拉变换的本质 ,减少了教学难
5) irrational number e


无理数e
1.
The paper points out the equivalence proprty of six formulas that have relation with irrational number e and proves them.
指出与无理数e有关的六个式子的等价关系并予以证明。
补充资料:无理数
| 无理数 irrational number 无限不循环十进小数 。例如 1.010010001… ,圆周率π=3.14159…等 。无理数是由于人们度量线段长度的需要而产生的,大约在2000年前,古希腊人发现以一个正方形的边为长度单位去量这个正方形的对角线,对角线的长度不能用有理数表示。原因是,根据勾股定理,对角线长度l必须满足l2=12+12,即l2=2。但又能证明了任何一个有理数的平方都不等于 2,从而证明了没有一个有理数能表示对角线的长度。为了使任意线段的长度都能用数表示,只好引进一种新的数,即无限不循环的十进小数,并称为无理数。表示上述正方形对角线长的数是一个无理数,用符号表示为 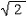 ,其值等于1.414213…。 ,其值等于1.414213…。 |
说明:补充资料仅用于学习参考,请勿用于其它任何用途。
参考词条