1) cardinal preferences


基数偏好
2) Preference function


偏好函数
1.
Preference Functions in Fuzzy Decision-Making Constructed with Close Degree;


利用贴近度构造模糊决策中的偏好函数
2.
Then,preference functions are developed and the design ranges and system ranges are identified.
该方法在决策时无需给出各评价指标的权重,只需决策者设定各指标满意区间的边界值,形成偏好函数,确定各指标的设计范围和系统范围,并通过计算产品各性能指标的信息量进行优劣排序,优选最佳产品。
3) preference parameter


偏好参数
1.
Thus,this paper quoted the preference parameter θ,in order to change the multiobjected problem into single-objected quadric planning problem.
为此引入偏好参数θ将多目标问题转化为单个目标的二次规划问题,既能弥补以前的模型的缺陷,又使得能寻找到更好的解法来获得有效投资组合。
4) preference number


偏好数
1.
Based on the principle of Arrow axiom, the group decision making rule is considered as social choice rule and the definition of group decision making rule based on preference number is proposed and several character conditions of Arrow rationality is discussed.
根据Arrow公理的基本原理 ,将群体决策规则作为社会选择准则进行研究 ,并基于群体偏好数的基本思路和方法 ,定义了偏好数群体决策规则 ,给出了合理选择规则的特征条件 。
5) preference coefficient


偏好系数
1.
Among models for investing combinatory,this model with preference coefficient is one of the most important models.
在投资组合各种模型中,具有偏好系数的投资组合模型是最重要的模型之一,对其求解的研究具有理论价值。
6) ordinal preference


序数偏好
1.
Dynamic programming approach to multi-attribute group decision-making with ordinal preferences
序数偏好下多属性群决策的动态规划法
2.
TOPSIS algorithm for multi-attribute group decision-making with weighted ordinal preferences
加权序数偏好下多属性群决策的TOPSIS法
补充资料:基数
| 基数 cardinal number 集合论中刻画任意集合所含元素数量多少的一个概念。又称势。两个能够建立元素间一一对应的集合称为互相对等集合。例如3个人的集合和3匹马的集合可以建立一 一对应,是两个对等的集合。根据对等这种关系对集合进行分类,凡是互相对等的集合就划入同一类。这样,每一个集合都被划入了某一类 。任意一个集合A所属的类就称为集合A的基数,记作 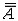 (或|A|,或cardA)。这样,当A 与B同属一个类时,A与B 就有相同的基数,即 (或|A|,或cardA)。这样,当A 与B同属一个类时,A与B 就有相同的基数,即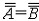 。而当 A与B不同属一个类时,它们的基数也不同。即 。而当 A与B不同属一个类时,它们的基数也不同。即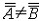 。 如果把单元素集的基数记作1,两个元素的集合的基数记作2,等等,则任一个有限集的基数就与通常意义下的自然数一致 。空集 。 如果把单元素集的基数记作1,两个元素的集合的基数记作2,等等,则任一个有限集的基数就与通常意义下的自然数一致 。空集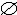 的基数也记作σ 。于是有限集的基数也就是传统概念下的“个数”。但是,对于无穷集,传统概念没有个数,而按基数概念,无穷集也有基数,例如,任一可数集与自然数集N有相同的基数 的基数也记作σ 。于是有限集的基数也就是传统概念下的“个数”。但是,对于无穷集,传统概念没有个数,而按基数概念,无穷集也有基数,例如,任一可数集与自然数集N有相同的基数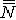 ,即所有可数集是等基数集。不但如此,还可以证明实数集R与可数集的基数不同,即 ,即所有可数集是等基数集。不但如此,还可以证明实数集R与可数集的基数不同,即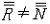 。所以集合的基数是个数概念的推广。基数可以比较大小。假设A,B的基数分别是a,β,即 。所以集合的基数是个数概念的推广。基数可以比较大小。假设A,B的基数分别是a,β,即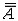 =a, =a,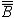 =β,如果A与B的某个子集对等,就称 A 的基数不大于B的基数,记作a≤β,或β≥a。如果 a≤ β,但a≠β( 即A与B不对等 ),就称A的基数小于B的基数,记作a<β,或β>a。基数可以进行运算 。设 =β,如果A与B的某个子集对等,就称 A 的基数不大于B的基数,记作a≤β,或β≥a。如果 a≤ β,但a≠β( 即A与B不对等 ),就称A的基数小于B的基数,记作a<β,或β>a。基数可以进行运算 。设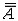 =a , =a ,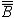 =β,且 A∩B= =β,且 A∩B=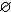 ,则规定 ,则规定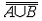 为a 与β之和记作 为a 与β之和记作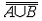 =a +β。设 =a +β。设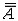 =a, =a,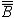 =β,A×B为 A与B的积集,规定 =β,A×B为 A与B的积集,规定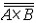 为 a 与β的积,记作 为 a 与β的积,记作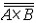 =a·β。 =a·β。 |
说明:补充资料仅用于学习参考,请勿用于其它任何用途。
参考词条