2) parabolic problem


抛物型问题
1.
The property of the lumped mass finite element method(LMFEM) on rectangular mesh is investigated to solve a typical parabolic problem,i.
就一类典型的抛物型问题——热传导方程,研究矩形网格上质量集中有限元方法的有关性质。
4) Exterior parabolic problem


抛物型外问题
5) parabolic obstacle problem


抛物型障碍问题
1.
In this paper, we have studied that the following form of a parabolic obstacle problem exist solution.
本文研究一个如下形式的抛物型障碍问题:求u(z,(?)),使得其中r、σ、μ、Κ、γ、l和L均为正常数,c和d是常数,满足c<lnΚ、d>lnΚ,ξ(z)是给定的已知函数,满足ξ∈C~∞([c,d]),ξ(z)<0于[c,d]且其中0<ν<d-lnΚ。
6) parabolic problem


抛物问题
1.
Rectangular finite element method with moving grid for parabolic problem;


抛物问题的一类变网格矩形有限元方法
2.
Finite Element Analysis of Parabolic Problem in the Domain with Curved Boundaries;


曲边区域上抛物问题的有限元分析
3.
This paper proposed a cascadic multigrid(CMG) method for parabolic problems in domains with re-entrant corners,whose corresponding convergent results were obtained.
针对凹角域上的抛物问题提出了瀑布型多重网格方法,获得了相应的收敛性结果。
补充资料:抛物型偏微分方程
| 抛物型偏微分方程 parabolic type,partial differential equation of 偏微分方程的一类。最典型的是热传导方程 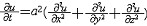 (a>0) (1)基本解是点热源的影响函数。若在t=0时在(ξ,η,ζ)处给定单位点热源,即u0(x0,y0,z0,0)=δ(ξ,η,ζ)(δ为狄拉克函数),则当t>0时便引起在R3的温度分布,这就是基本解。用傅里叶变换可得到它的表达式 (a>0) (1)基本解是点热源的影响函数。若在t=0时在(ξ,η,ζ)处给定单位点热源,即u0(x0,y0,z0,0)=δ(ξ,η,ζ)(δ为狄拉克函数),则当t>0时便引起在R3的温度分布,这就是基本解。用傅里叶变换可得到它的表达式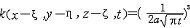 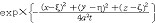 热传导方程初值问题的解可用基本解叠加而成,即 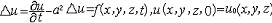 的解为 的解为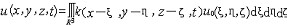 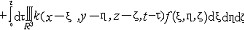 极值原理:一个内部有热源的传导过程,它的最低温度一定在边界上或初始时刻达到。更强的结论是 :如果t=T时在Ω内某一点达到最低温度 ,则在这个时刻以前(t<T时)u≡常数 ;又:若最低温度在t=T时边界¶Ω上某点P达到,则在这点上 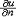 |P,Τ<0(n为外法线方向)。 |P,Τ<0(n为外法线方向)。 |
说明:补充资料仅用于学习参考,请勿用于其它任何用途。
参考词条