1) technics of least squares
最小二乘方术;最小平方技术
2) least-squares technique
最小二乘技术
1.
Satisfaction of specified boundary conditions can produce the final linear system of equations,which is solved by the simple least-squares technique and truncated singular value decomposition(TSVD) to determine all unknown interpolation coefficients,and then temperature field at arbitrary points can be obtained.
最小二乘技术和截断的奇异值分解方法被用来对方程组进行对称化处理和求解并确定所有未知插值系数,进而计算出域内任意位置的温度分布。
3) method of least squares
最小二乘法;最小平方法
4) least square
最小二乘方
1.
A new method of least square is raised in curve fitting of flood frequency calculating and its specific properties are analysed.
提出了在洪水频率计算时适线法中最小二乘方的一种新的求解方法 ,并对其特性进行分析。
2.
In this paper,real-time separation is conducted using the principal of least square as well as the fact that the speech signal is non-stationary.
在本文中,利用最小二乘方原理并结合语音信号非平稳的特点,对混合语音信号进行实时分离。
5) least square
最小二方乘
6) moving least squares technique
滑动最小二乘技术
补充资料：最小二乘法
| 最小二乘法 least square，method of 科学实验和统计工作中常用的一种数据处理方法。由A.M.勒让德和C.F.高斯于19世纪初分别独立提出。例如要从一组实验数据（xi，yi）（i=1，2，…，m）中，寻找自变量x与因变量y之间的函数关系y=F（x）。由于观测数据往往不准确，因率不要求y=F（x）经过所有数据点，而只要求所在所有给定点xi上的偏差ri＝F（xi）－yi（i＝1，2，…，m）的平方和 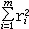 达到最小。F（x）的函数类型往往与实验的物理背景以及数据的实际分布有关，它一般含有某些待定参数。如果F（x）是所有待定参数的线性函数，那么相应的问题称为线性最小二乘问题，否则称为非线性最小二乘问题。最小二乘法还是实验数据参数估计的重要工具。这是因为这种方法比其他方法更容易理解，即使在其他方法失效的情况下，用最小二乘法还能提供解答，而且从统计学的观点分析，用该方法求得各项估计具有最优统计特征，因此这一方法也是系统识别的重要基础。线性最小二乘问题可以借助多元微分学知识通过求解法方程组得到解答。求解非线性最小二乘问题比较困难，一般要用线性化方法或最优化方法才行。 |
说明：补充资料仅用于学习参考，请勿用于其它任何用途。
参考词条