1) the set of all odd numbers


奇数集
2) dimension of singular set


奇异集维数
3) singular set function


奇异集函数
4) singular additive set function


奇异加性集函数
5) Odd number


奇数
1.
Let p>q and q be an odd number,We discuss the condition of no positive integer solution for the Generalized Fermat equation x~p+y~q=z~q.
当p>q,且q为奇数时,探讨广义Fermat方程xp+yq=zq无正整数解的条件,并提出一个猜想。
2.
By the resolution of a mathematical problem,this paper carries out a further research of using property of the even number and odd numbers to get the relevant theorem of resolve this problem,and explain concrete application by an example.
通过一个数学问题的解决,由此提出了进一步研究的问题,利用奇数与偶数的有关性质,得到了解决这一类问题的有关定理,并且通过例子说明了定理的具体应用。
3.
Here r is not a negative whole number,h,x are odd numbers and h>0 .


断定,当n=2r+1 -1时,若{x+1}2 =m,那么对于s(x) =∑ni=0xi就有{s(x)2 } =m+r成立,此处r是非负整数,x≠±1;当n=2r+1h-1时,若{x+1}2 =m,那么对于s(x) =∑nxi就有{s(x) } =m+r成立,此处r是非负整数,h,x为奇数,且h>0。
补充资料:奇异数
| 奇异数 strangeness 描述粒子内部性质的一种相加性量子数 。记为S。1947年观测到一起新奇的粒子反应,反应中生成的新粒子的性质与以前发现的粒子不同 ,有一些新奇的特征。它们产生快、衰变慢,它们协同产生、单独衰变,以后又发现一些其他类似的粒子。通过分析研究弄清楚它们的产生过程属于强作用,衰变过程属于弱作用。协同产生表明产生过程有某种限制,这一特征引入奇异数S来区分这些新奇的粒子和以前发现的粒子,例如p、n、π±、π0、μ-、μ+、e-、e+、γ等S=0,K0、K+的S=+1, 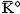 、K-、Λ0、Σ±、Σ0的S=-1,Ξ0、Ξ-的S=-2,Ω-的S=-3。反粒子的奇异数与粒子的奇异数符号相反。在强作用和电磁作用过程中,奇异数守恒;在弱作用过程中,奇异数可以不守恒,ΔS=0,±1。 、K-、Λ0、Σ±、Σ0的S=-1,Ξ0、Ξ-的S=-2,Ω-的S=-3。反粒子的奇异数与粒子的奇异数符号相反。在强作用和电磁作用过程中,奇异数守恒;在弱作用过程中,奇异数可以不守恒,ΔS=0,±1。 |
说明:补充资料仅用于学习参考,请勿用于其它任何用途。
参考词条