1) Simple friction problem
简单摩擦问题
2) Friction problem
摩擦问题
1.
MRM-boundary mixed variational inequality of the second kind in friction problem;
摩擦问题中的第二类MRM-边界混合变分不等式
2.
Friction problem can be formulated as a nonlinear variational inequality.
摩擦问题可表述为第二类非线性变分不等式，笔者给出了对此问题在多子域情形下的Schwarz算法，并证明了此算法的收敛性。
3.
Using the friction problem in elasticity as the background,the mixed varia- tional inequality of the second kind in friction problem is reduced to an MRM-boundary mixed variational inequality by the multiple reciprocity method(MRM).
本文以弹性力学中的摩擦问题为背景,采用多重互易方法(MRM方法),边界元方法,将摩擦问题中的第二类混合变分不等式化解为MRM-边界混合变分不等式,给出了MRM-边界混合变分不等式解的存在唯—性,通过引入变换将原MRM-边界混合变分不等式化解为标准的凸极值问题,采用正则化方法处理后,给出了MRM-边界混合变分不等式的迭代分解方法。
3) frictional contact problems
接触摩擦问题
1.
Numerical modeling for frictional contact problems;
接触摩擦问题的数值模拟
4) frictional contact problems
摩擦接触问题
1.
The multiple nonlinear properties of three dimensional frictional contact problems make its calculation difficult.
三维摩擦接触问题具有多重非线性性质,使求解变得比较困难,为了解决此问题,建立了三维摩擦接触问题的模型,介绍了一种非光滑混合不动点算法。
2.
The multiple nonlinear properties of three-dimensional frictional contact problems make its calculation difficult.
摩擦接触现象在工程实际及日常生活中到处可见，三维摩擦接触问题具有多重非线性性质，使求解变得比较困难，目前有很多种求解摩擦接触问题的方法，在实际工程计算中得到广泛应用的是迭代法，该方法过程简单、易于实施，但是其收敛性不能保证，建立既准确又保证收敛的求解摩擦接触问题的高效方法一直是一个难题。
3.
Based on this,a parametric augmented Lagrangian quadratic programming algorithm was established for the frictional contact problems.
建立了摩擦接触条件的增广Lagrange线性互补表述形式,继而构造出求解摩擦接触问题的一种增广Lagrange线性互补算法。
5) the problem of the static indeterminate with friction
有摩擦的超静定问题
6) viscoelastic contact with friction
粘弹性摩擦接触问题
1.
A kind of evolutionary variational inequality arising in the problem of viscoelastic contact with friction
一类粘弹性摩擦接触问题中的发展型变分不等式
2.
The problems of viscoelastic contact with friction are extensively applied in many fields such as physics, mechanics, mechanical engineering etc.
粘弹性摩擦接触问题在物理、力学、机械工程等众多领域有着广泛应用。
补充资料：摩擦学:滚动摩擦
滚动摩擦
两相互接触的物体有相对滚动或有相对滚动趋势时的摩擦。滚动摩擦的机理不同於滑动摩擦﹐它主要来源於滚动体在接触区域產生弹-塑性变形﹐在以接触点(或线)为中心的接触区内接触压力分布不对称﹐致使支承面的反力N 发生偏心﹐并產生摩擦力F (见图 滚动摩擦示意图
)。用表示法向反力N 到接触点的距离﹐根据力的平衡条件可得
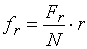
式中为滚动体半径﹔为滚动摩擦係数﹐它的量纲为长度﹐其值与接触面的材料﹑粗糙度﹑温度和湿度等因素有关。
两相互接触的物体有相对滚动或有相对滚动趋势时的摩擦。滚动摩擦的机理不同於滑动摩擦﹐它主要来源於滚动体在接触区域產生弹-塑性变形﹐在以接触点(或线)为中心的接触区内接触压力分布不对称﹐致使支承面的反力N 发生偏心﹐并產生摩擦力F (见图 滚动摩擦示意图
)。用表示法向反力N 到接触点的距离﹐根据力的平衡条件可得 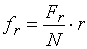式中为滚动体半径﹔为滚动摩擦係数﹐它的量纲为长度﹐其值与接触面的材料﹑粗糙度﹑温度和湿度等因素有关。
说明：补充资料仅用于学习参考，请勿用于其它任何用途。
参考词条