1) the Ampere Circuit Theorem of Universal Suitable Type


普适型安培环路定理
1.
It dissects the existing abuses of the original theorem in the object use and then proceed to putting forward an improved"the Ampere Circuit Theorem of Universal Suitable Type".
从学生在应用"安培环路定理"时所犯的一个普遍性的错误着手,分析了产生错误的主、客观原因,剖析了原定理在直观应用上所存在的弊端,进而给出了一个改进的"普适型安培环路定理"。
2) Ampere circuit rule


安培环路定理
1.
A new method to demonstrate the Ampere circuit rule;


安培环路定理的一种证明方法
2.
The Ampere circuit rule in steady magnetic field is demonstrated generally, avoiding vector analysis.
利用磁壳与线形闭合电流等效的证明方法,可避开使用矢量分析工具,一般地证明稳恒磁场安培环路定理。
3) A deduction to the Ampere circuital theorem


推证安培环路定理
4) Ampere circuit theorem


安培环路定律
1.
Applying this method into teaching,it will enhance the understanding of Ampere circuit theorem and make the undergraduates master the application of this theorem in practice,stimulate the studying interests and improve the ability of integrating theory with practice.
本文把一个抽象的电磁场定律具体化,阐述了安培环路定律在磁场屏蔽工程实践中的应用。
5) Ampere ring road


安培环路
1.
The methods of choosing Ampere ring road and the applicat io n of Ampere ring road theorem to the solution of magnetic field conditions are b oth presented in detail,concerning the calculation of magnetic induction streng th within spiral winded wreath.
针对铁芯有缝的螺绕环内磁感应强度的计算,阐明安培环路的选择及应用安培环路定理求解磁场的条件。
6) Ampere's theorem


安培定理
补充资料:安培环路定理
| 安培环路定理 Ampere's closed loop,theorem of 关于恒定磁场性质的一个基本定理。恒定磁场的磁感应强度B沿任意闭合路径l 的线积分,等于穿过l 所围曲面(l )的电流强度的代数和的μ0倍(μ0为真空磁导率),即 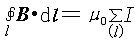 式中电流I的正负号规定为:当穿过(l)的电流方向与积分的环绕方向构成右手螺旋关系时I取正,反之,I取负。如果电流不穿过(l),虽然它产生的磁场对B有贡献,但B的环路积分为零。 式中电流I的正负号规定为:当穿过(l)的电流方向与积分的环绕方向构成右手螺旋关系时I取正,反之,I取负。如果电流不穿过(l),虽然它产生的磁场对B有贡献,但B的环路积分为零。若存在磁介质,磁化后出现磁化电流I′,上式的I是传导电流I0和I'之和。由于I'未知,为了使上式不显现I',引入磁场强度 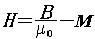 (M是磁介质的磁化强度),把有磁介质存在时的安培环路定理表为 (M是磁介质的磁化强度),把有磁介质存在时的安培环路定理表为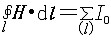 对于线性各向同性磁介质,B=μrμ0H,μr是磁介质的相对磁导率(见磁导率)。 对于线性各向同性磁介质,B=μrμ0H,μr是磁介质的相对磁导率(见磁导率)。恒定磁场的安培环路定理可根据毕-萨-拉定律及磁场叠加原理证明。它表明磁场是有旋的,闭合的磁力线与载流回路相互套连。利用安培环路定理可以计算某些对称分布电流产生的磁场。它还是磁路设计的理论基础。 在非恒定情形,如果既有传导电流又有位移电流,J.C.麦克斯韦把安培环路定理的右端推广为包括位移电流在内的全电流,即 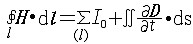 式中D是电位移; 式中D是电位移;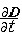 是位移电流密度。推广的安培环路定理是麦克斯韦方程组的一个方程,它是麦克斯韦在建立电磁场理论过程中作出的大胆假设和主要贡献。 是位移电流密度。推广的安培环路定理是麦克斯韦方程组的一个方程,它是麦克斯韦在建立电磁场理论过程中作出的大胆假设和主要贡献。 |
说明:补充资料仅用于学习参考,请勿用于其它任何用途。
参考词条