1) the Fuzzy numerical cotangent function


模糊数值余切函数
2) Fuzzy number-valued cosine function


模糊数值余弦函数
3) the Fuzzy numerical tangent function


模糊数值正切函数
1.
This paper discusses the Fuzzy numerical tangent function and Fuzzy numerical cotangent function based on the extension theory,and further studies their basic characteristics.
利用扩展原理引入了模糊数值正切函数与余切函数 ,并研究了这两种模糊函数的基本性
4) fuzzy-valued function


模糊值函数
1.
Integral and requirement of fuzzy-valued function;


模糊值函数的积分及可积条件
2.
Convergence and continuity of fuzzy-valued functions;


模糊值函数的收敛性及连续性
3.
Linear representation of fuzzy number and fuzzy-valued function using fuzzy structured element;
模糊数与模糊值函数的结构元线性表示
5) fuzzy-valued functions


模糊数值函数
1.
The Differentiability of Primitives for the Fuzzy-Valued Functions;


模糊数值函数积分原函数的可导性问题
6) series of fuzzy valued functions


模糊值函数级数
1.
The absolute uniform convergence for the series of fuzzy valued functions;


模糊值函数级数的绝对一致收敛性
补充资料:余切函数
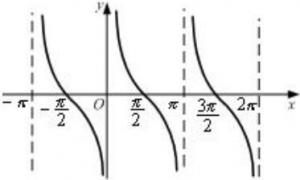
在y=ctgx中,以x的任一使ctgx有意义的值与它对应的y值作为(x,y),在直角坐标系中,作出y=ctgx的图形叫余切函数图象.也叫余切曲线.如图3-8.它是由相互平行的x=kπ(k∈z)直线隔开的无穷多支曲线所组成的.
余切函数,y=cotα=x/y,余切值=邻边/对边.
y=ctgx的性质:
(1)定义域x≠kπ(k∈z)
(2)值域y∈r,当x→2kπ时,y→∞;当x→(2k+1)π时,y→-∞.
(3)y=ctgx是奇函数,即ctg(-x)=-ctgx,图象对称于原点.
(4)y=ctgx是周期函数,周期为kπ(k∈z且k≠0),最小正周期t=π.
(5)y=ctgx在每一个开区间(kπ,(k+1)π)(k∈z)内部是减函数.
把正切图像向左平移∏/2,然后把x和-x互换就可以得到余切函数的图象,也就是说ctgx=tg(-x+∏/2).性质和正切函数的性质完全一样!
说明:补充资料仅用于学习参考,请勿用于其它任何用途。