1) C-complement topological space
c-补拓扑空间
1.
On the basis of limitary complement topological space and countable complement topological space,the concept of C-complement topological space is then introduced.
本文首先给出了c-集的概念,然后在有限补拓扑空间及可数补拓扑空间的基础上引入了c-补拓扑空间的概念并对其性质进行了初步探讨。
2) topologically complemented subspace
拓扑可补子空间
3) countable complementary topological space
可数补拓扑空间
4) topological spaces
拓扑空间
1.
Fractal dimensions of polyferric chloride-humic acid(PFC-HA) flocs in different topological spaces;
聚合氯化铁-腐殖酸(PFC-HA)絮体的不同拓扑空间下分形维数的研究
2.
A semigroup of closed selfmaps of a kind of topological spaces;
一类拓扑空间的闭自映射半群
3.
The physical properties and fractal dimensions within different topological spaces of the mature granular sludge in an anaerobic baffled reactor (ABR) were investigated.
研究了ABR反应器启动成功后成熟颗粒污泥的物理性质和不同拓扑空间下的分形维数。
5) topological space
拓扑空间
1.
Seven definitions of topological space and their sameness;
拓扑空间的七个定义及其等价性
2.
Some Browder type fixed point theorems in topological spaces with applications;
拓扑空间中的Browder型不动点定理及应用
3.
Some properties of the relative topological space;
相对拓扑空间的一些性质
6) topology space
拓扑空间
1.
In reference 1 ,the theorems about fibre boundness and compactness of uniform space with shadow to be topology space were given.
文献〔１〕，给出了像为拓扑空间Ｔ的一致空间Ｘ的纤维有界性、纤维紧致性的一些定理。
2.
A cover U of a topology space X has a alternate σ-relatively locally finite and relatively closed refinement.
称拓扑空间X的开覆盖U有迭次σ-相对局部有限相对闭加细,如果U有一个加细PP(n,k)满足:(1) n,k∈N+,P(n,k)相对子空间X-∪P*(i)∪∪P*(n,j),∪∞=∪∞n=1k=1i
补充资料：拓扑空间
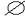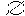| 拓扑空间 topological space 赋予拓扑结构的集合。如果对一个非空集合X给予适当的结构，使之能引入微积分中的极限和连续的概念，这样的结构就称为拓扑，具有拓扑结构的空间称为拓扑空间。引入拓扑结构的方法有多种，如邻域系、开集系、闭集系、闭包系、内部系等不同方法。下面介绍开集系方法。在微积分学中，实一维欧几里得空间R′上的开集具有性质：①任意个开集的并是开集 。②有限个开集的交是开集。③R′及空集  是开集。对任一非空集合X，若X的一个子集族J满足：①J中元的任意并在J中。②J中元的有限交在J中。③X、 在J中，则称J是X的一个拓扑，J中的元称为开集，X连同拓扑J称为一个拓扑空间，记为（X，J）。对任意x∈X，如果Z的子集U包含含有x的一个开集则U称为x的一个邻域。如果X的子集A满足X－A是开集，则称X是闭集。 设X是非空集合，令J0＝｛X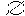，  ｝，称（X，J0）为平庸拓扑空间，J0为平庸拓扑。令J1＝｛A｜AÌX｝，称（X，J1）为离散拓扑空间。在离散拓扑空间中任意子集均是开集。对实数集R1，令J＝｛BÌR1｜"x∈G，∈ε＞0，使（x－ε，x＋ε）ÌG｝，则（R1，J）就是一维欧几里得空间。类似地可定义n维欧几里得空间Rn。设X是拓扑空间，如果X可写为非空开集的分离并，则X称为连通空间；如果对X中任意两点 ，存在X中的道路相连接，则称X为道路连通空间 ；如果X的任意开集作成的覆盖存在有限子覆盖 ，则称X为紧空间；如果X中的任意序列有收敛子列，则称X是列紧空间 ；如果X中任意两点都存在不相交的邻域 ，则称X是豪斯多夫空间（或T2空间）。上面所提连通性，道路连通性、紧性、列紧性、T2性均是拓扑不变性。连通空间上的实值连续函数具有介值性，即若f∶X→R1连续，X是连通空间，r∈（f（x1），f（x2），则存在c∈（x1，x2）（或c∈（x2，x1）），使f（c）＝r。紧空间上的实值连续函数具有最大值、最小值。紧空间上的连续函数一致连续。若AÌRn，则A为紧，当且仅当A是有界闭集。 |
说明：补充资料仅用于学习参考，请勿用于其它任何用途。
参考词条