1) linear vibration
线性振动
1.
A new method is suggested to derive the Duhamel integral in the linear vibration systems of singledegreeof freedom for the response to arbitrary excitations by the depression of order of integral factor.
利用积分因子降阶法，给出了确定单自由度线性振动系统对任意激励响应的杜哈梅积分的一种新的推导方法。
2.
This paper investigates robust H ∞ control for a linear vibration system with uncertain parameters.
研究了含有参数不确定性的线性振动系统的鲁棒Ｈ∞控制，折衷考虑振动系统的外激励干扰衰减和振动系统参数摄动的鲁棒性问题。
3.
This paper deals with the solution method of the initial value problem of linear vibration systems in the time domain.
研究线性振动系统初值问题的时域求解方法。
2) nonlinear vibration
非线性振动
1.
Homotopic perturbation method for nonlinear vibrations of bimetallic shallow shells of revolution;
双层旋转扁壳非线性振动分析的同伦摄动法
2.
Adaptive fuzzy sliding mode control for nonlinear vibration reduction of structure;
结构非线性振动的自适应模糊滑模控制
3.
Combination resonance of laterally nonlinear vibration of axially moving systems;
轴向运动体系横向非线性振动的联合共振
3) non-linear vibration
非线性振动
1.
Study and application of non-linear vibration theory in vibratory conveyer;
非线性振动理论在振动输送机中的研究与应用
2.
Compare the results of two methods of non-linear vibration of a spur gear pair;
直齿轮副非线性振动分析中两种解法的结果比较
3.
The non-linear vibration in two-dimension of three flexible symmetric simple harmonic oscillator;
对称四弹性振子的二维非线性振动
4) nonlinear oscillation
非线性振动
1.
On the nonlinear oscillation of internal sliding friction in particulate matter;
颗粒物质内部滑动摩擦力的非线性振动现象
2.
Establishment and Approximate Solution to Horizontal Equation in Case of Nonlinear Oscillation of Plane Pendelum;
平面摆非线性振动水平分量方程的建立及其近似解
3.
By using averaging method for nonlinear oscillation, internal resonance, primary resonance,and double resonances of the first order approximation solutions and their corresponding steady state solutions of the system are obtained.
应用非线性振动的平均法,求得了系统满足3∶1型内共振、主共振及双重共振条件的解。
5) linear shale shaker
线性振动筛
1.
Development of model ZS6 drllimg fluid linear shale shaker;
ZS6型钻井液线性振动筛研制
6) nonlinear oscillations
非线性振动
1.
ontrolling chaos is surveyed as a new direction in nonlinear oscillations and vibration control in this paper.
概述了近年来在控制混沌这一非线性振动和振动控制新的研究方向上的进展，首先介绍了控制混沌的内容和意义，然后阐述了控制混沌的几类主要方法，包括输送控制、镇定控制和非线性系统理论应用的原理和发展。
2.
This paper begins with surveying the progress madein the new research field of chaos control in nonlinear oscillations.
综述了控制非线性振动中混沌这一新研究领域的若干进展，即非线性振动中混沌的抑制、非线性振动的输送控制、混沌吸引子中不稳定周期性轨道的稳化、随机控制、自适应控制及简单反馈控制在控制混沌中的应用，指出了各种方法的原理、应用、特点和局限制。
3.
The nonlinear oscillations of fluid conveying pipes under parametric and external excitations were researched.
研究了参数激励和外激励联合作用下输流管道的非线性振动问题。
补充资料：线性振动
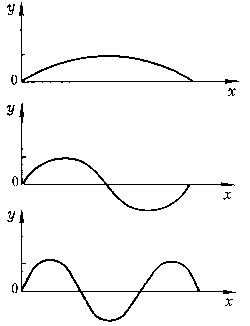
| 线性振动 linearvibration 系统中构件的弹性服从胡克定律，运动时产生的阻尼力与广义速度（广义坐标的时间导数）的一次式成正比的振动。它通常是实际系统微幅振动的一个抽象模型。线性振动系统适用叠加原理，即如果在输入x1作用下，系统响应为y1，而在输入x2作用下，系统响应为y2，则系统在输入x1和x2的联合作用下的响应就是y1＋y2。在叠加原理基础上，可把一个任意的输入分解为一系列微元冲量的和，然后求得系统的总响应；还可将一个周期激励经傅里叶变换，展成一系列谐和分量之和，分别考察各谐和分量对系统的作用结果，再将它们叠加起来，就得到系统的总响应。因此，常参量线性系统的响应特性可用脉冲响应或频率响应描述。脉冲响应指系统对单位冲量的响应，表征系统在时域内的响应特性。频率响应指系统对单位谐和输入的响应特性，表征系统在频域内的响应特性。两者由傅里叶变换确定对应关系。 单自由度系统的线性振动是可用一个广义坐标来确定系统位置的线性振动。它是最简单的振动，许多振动的基本概念和特征可由此引出。它包括简谐振动、自由振动、衰减振动和受迫振动。 多自由度系统的线性振动是自由度n≥2的线性系统的振动。图1
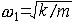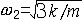 , ，每个频率对应一种振动形态。各简谐振子进行同频率的谐和振动，同步地通过平衡位置，又同步地到达极端位置，这种振动称为主振动。在对应于ω1的主振动中，有x1＝x2；在对应于ω2的主振动中，有x1＝－x2 。在主振动中各质量的位移之比保持一个确定的关系，构成一个确定的振型，称为主振型或固有振型。各主振型之间存在着关于质量与刚度的正交性，它反映各主振动之间的相互独立性。固有频率与主振型表征多自由度系统固有的振动特性。一个n自由度系统有n个固有频率和n个主振型。系统的任何振动形态都可以表示成各个主振型的线性组合。因此，在多自由度系统动态响应分析中，广泛采用主振型叠加法。于是，系统固有振动特性的测试和分析也就成为系统动态设计的一个常规步骤。多自由度系统的动态特性也可以用频率响应描述。由于各输入输出之间都有一个频率响应函数，从而构成一个频率响应矩阵。频率响应与主振型之间有确定的关系。和单自由度系统不同，多自由度系统的幅频特性曲线有多个共振峰。弹性体的线性振动是弹性体有无限多个自由度，因而具有无限多个固有频率和无限多个主振型。弹性体的任何振动形态也可表示为各主振型的线性叠加。因而对于弹性体的动态响应分析，主振型叠加法仍然适用。以弦的振动为例。设单位长度质量为m的细弦，长l，两端张紧 ，张力为T。弦的固有频率fn＝na／2l（n＝1，2，3，…），式中a＝（T／m）1/2，是横波沿弦线方向的传播速度。弦的各阶固有频率恰巧为基频a／2l的整数倍 。这种整数倍关系导致悦耳的谐音结构。一般弹性体各阶固有频率并不存在这种整数倍关系。张紧弦的前三阶振型如图2
所示。取一弦端为x轴原点 ，则对应第n阶固有频率fn的主振型为yn（x）＝Asin（nπx／l），式中A为振幅。主振型曲线上有一些节点，弦的第n阶主振型有n－1个节点。在主振动中，各节点不振动。弹性体的线性振动在数学上可归结为偏微分方程的边值问题。但只有在一些最简单的情况下才能找到准确解，因而对于复杂的弹性体的线性振动问题必须求助于近似解法。各种近似解法的要旨是变无限为有限，也就是将无限多自由度系统（连续系统）离散化为有限多个自由度系统（离散系统）。工程分析中广泛采用的离散化方法有两大类：有限元法与模态综合法。 |
说明：补充资料仅用于学习参考，请勿用于其它任何用途。
参考词条